Social distancing is integral to our lives these days, but distancing also underpins the ordered patterns and arrangements we see all around us in Nature. Oxford Mathematician Priya Subramanian studies the defects in such patterns and shows how they relate to the underlying pattern, i.e. to the distancing itself.
"Nature has many examples of situations where individuals in a dense group have to balance between short range repulsion (e.g., competition for resources) and long range attraction (e.g. safety in numbers). This naturally leads to an optimal length scale for separation between neighbours. If we think of each agent as a circle with this optimal length as the diameter, the most efficient packing is in a hexagonal arrangement. So, it is unsurprising that we find these group of gannets nesting on a beach in Muriwai, New Zealand forming a largely hexagonal pattern.
Figure 1: When neighbours keep their distance: gannets nesting at Muriwai Beach, New Zealand. The image on the left shows the overall hexagonal ordering of the birds while that on the right shows a penta-hepta defect (PHD). The green (pink) markers identify gannets with five (seven) neighbours instead of the usual six. Photo credit: Barbora Knobloch. Adapted from [1].
Patterns are rarely perfect and defects arise due to many factors such as boundary conditions (e.g. cliff edge), fluctuations in the background (e.g. unevenness in the ground), etc. A generic defect that arises in such hexagonal patterns is highlighted in the right panel of the picture of the gannets. Normally each gannet will have six neighbours, but here we see that the gannet marked with a green dot has only five neighbours while the gannet marked with a pink marker has seven. Such a structure consisting of a bound state with one location having five neighbours and another location seven neighbours, instead of the usual six, is called a penta-hepta defect (PHD). Hexagonal arrangements are found in many areas of physics, from patterns formed in heated fluids, to self-assembled crystals formed in both hard materials (e.g. graphene) and soft materials (e.g. star-shaped polymers [2]). Equally prevalent in all such hexagonal arrangements is the possibility for PHDs.
Traditionally pattern formation techniques used to investigate defects use an amplitude-phase formulation, where a periodic pattern has a homogenous amplitude and a varying phase. The topological charge of a defect is calculated by integrating the phase around any closed curve enclosing the defect, and this quantity does not change when the defect moves. Topological defects [3] are associated with zeros of the amplitude (where the phase becomes undefined): these defects have non-zero topological charge and so they can only be eliminated or healed by interacting with another topological defect with opposite charge. On the other hand, non-topological defects, such as PHDs, have a well-defined phase everywhere (implying zero topological charge) and so were thought to be able to heal by themselves. However, if the defect has an internal structure it may persist as a result of frustration and get locked/pinned to the background periodic state: the gannets in the PHD in Figure 1 could re-arrange themselves to remove the defect, but to do so would involve all nearby gannets moving a little bit, so in practice they don't.
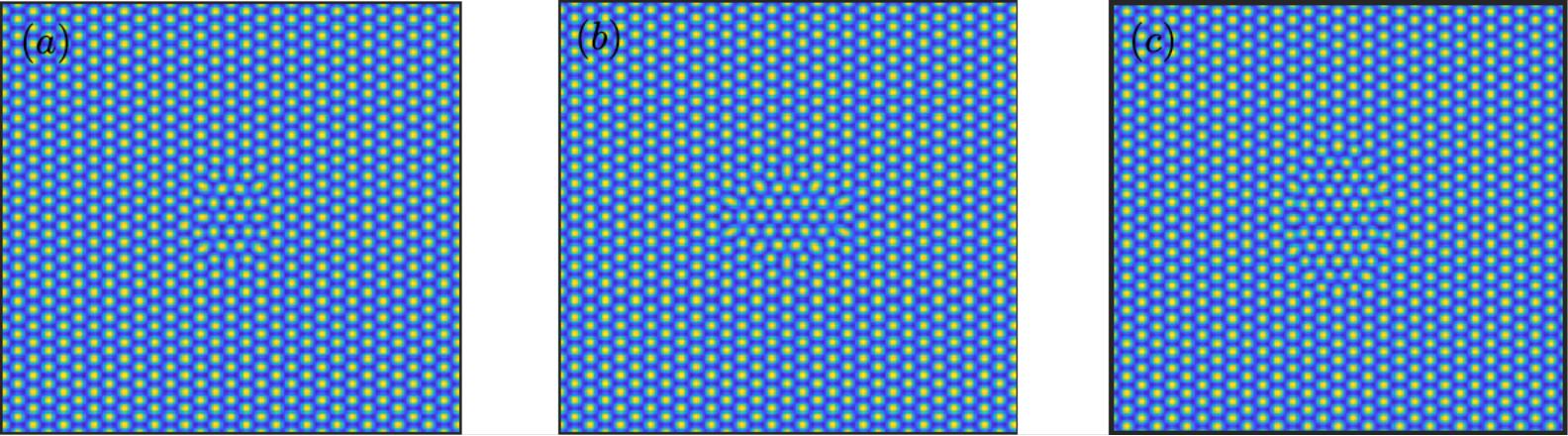
Figure 2: Coexisting equilibria with penta-hepta defects separating regions of hexagons with different orientations in the SH23 system [1]. All three states are dynamically metastable.
We explore such non-topological defects in the prototypical pattern forming Swift-Hohenberg model in our recent work [1], by adopting a different point of view and thinking of defects as spatially localised structures [4]. We focus on grain boundaries separating two-dimensional hexagon crystals at different orientations (shown in Figure 2): these grain boundaries are closed curves containing a ring of PHDs. Even with the parameters all the same, the model has many different stable configurations of these grain boundaries, and solution branches connected to each of these states form isolas that span a wide range of the model parameters, opening up multiple interesting questions about such defect states. Our results will also be applicable to understanding the role of grain boundaries in two dimensional solids such as graphene [5], in which defects play a crucial role near phase transition, i.e., melting [6], and in determining bulk properties of a material."
References: