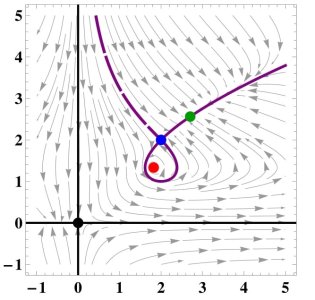
There is a wide class of problems in mathematics known as inverse problems. Rather than starting with a mathematical model and analysing its properties, mathematicians start with a set of properties and try to obtain mathematical models which display them. For example, in mathematical chemistry researchers try to construct chemical reaction systems that have certain predefined behaviours. From a mathematical point of view, this can be used to create simplified chemical systems that can be used as test problems for different mathematical fields. From an experimental perspective, it is useful to create chemical systems that can be used as blueprints for constructing physical networks in synthetic biology, for example in the area of DNA computing.
Oxford Mathematicians Tomislav Plesa and Radek Erban, together with their colleague Tomáš Vejchodský of the Institute of Mathematics in the Czech Academy of Sciences, have recently published a paper in the Journal of Mathematical Chemistry developing the theory behind this. They have built on previous work to create an inverse problem framework suitable for constructing a reaction system, and have used this to construct certain two- and three-dimensional systems of particular interest. Their work concentrates primarily on networks modelled by systems of kinetic equations, a class of ordinary differential equations of a certain type. Such equations may display 'exotic phenomena', corresponding to specific biological functions in the underlying biochemical reaction networks, and so the inverse problem framework needs to be able to handle such behaviours.