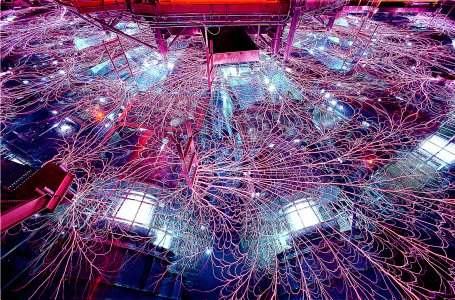
This picture shows the "Z" machine at Sandia Labs in New Mexico producing, for a tiny fraction of a second, 290 TW of power - about 100 times the average electricity consumption of the entire planet. This astonishing power is used to subject metal samples to enormous pressures up to 10 million atmospheres, causing them to undergo violent plastic deformation at velocities up to 10 km/s. How should such extreme behaviour be described mathematically?
Oxford DPhil student Stuart Thomson is working with Peter Howell, John Ockendon, Hilary Ockendon and collaborators at AWE to answer this question. To a first approximation, the plastically flowing metal behaves like a compressible inviscid gas, with small but important elastic waves superimposed. The team’s simulations and analysis explain and quantify the experimentally observed behaviour, and reveal a fascinating and previously unexplored phenomenon whereby fast-moving elastic waves reflect off slower-moving plastic waves. The results shed crucial light on the inverse problem of backing out the effective equation of state from the experimentally measured response of the sample, as well as posing fundamental theoretical questions about singularly perturbed hyperbolic systems.
This research is funded by an EPSRC Industrial CASE award through the Smith Institute for Industrial Mathematics and System Engineering.