Oxford Mathematician Kristian Kiradjiev has been awarded the Institute of Mathematics and its Applications (IMA) Early Career Mathematicians Catherine Richards Prize 2017 for his article on 'Exploring Steiner Chains with Möbius Transformations.' Here he explains his work.
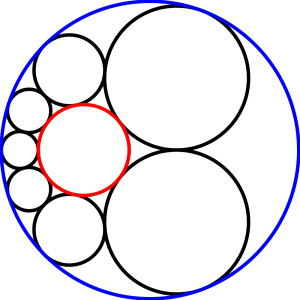
"A beautiful example that one encounters in the geometry of circles is the so-called Steiner chains, named after the Swiss mathematician Jacob Steiner. A Steiner chain is defined as a chain of $n$ circles, each tangent to the previous one and the next one, and also to two given non-intersecting circles, which we will call bounding circles. We focus exclusively on Steiner chains, one of whose bounding circles lies within the other, although the concept also exists when the bounding circles are disjoint. We also define a closed Steiner chain to be such that the first and last circles of the chain are tangent to each other. For the sake of simplicity, we will limit ourselves to simple closed chains, i.e. wrapping only once around the inner bounding circle, although, again, one can have multi-cyclic closed chains, which wrap around several times before touching the first circle.
One of the main results, concerning Steiner chains is known as Steiner's porism. A porism is a mathematical proposition, which nowadays usually refers to a statement that is either not true, or is true and holds for an infinite number of values, provided a certain condition is satisfied (cf. Poncelet's porism). Steiner's porism states that given two bounding circles and the first circle from the chain, if a Steiner chain exists, then there are infinitely many of them, irrespective of the position of the first circle.
A lot of other fascinating properties have been discovered. For example, it is known that the centres of the circles in the chain lie either on an ellipse (or circle) when one of the bounding circles lies within the other, or on a hyperbola if not. Also, the points of tangency between the circles in the chain happen to lie on a circle. More interestingly, using inversion (and the inversive distance invariant), a feasibility criterion has been established for whether a closed Steiner chain is supported for a given $n$ and a pair of bounding circles. The problem I considered is somewhat the opposite: given $n$ positive numbers, does there exist a pair of bounding circles such that we can arrange $n$ circles with radii the given $n$ numbers in a simple closed Steiner chain between these bounding circles? This can also be reformulated as a geometrical problem of inscribing and circumscribing circles around a chain of touching circles with given radii. In order to answer this question, I essentially relied on the concept of Möbius transformations, which are conformal maps (i.e. preserve angles) in the extended complex plane $\widetilde{\mathbb{C}}=\mathbb{C}\cup\{\infty\}$ of the form \begin{equation*} f(z)=\frac{az+b}{cz+d}, \label{eq:1} \end{equation*} where $z\in\widetilde{\mathbb{C}}$, and $a,b,c,d\in\mathbb{C}$ with $ad-bc\neq 0$ (so as to avoid constant maps). We note that translation, scaling, and inversion are particular cases of Möbius transformations. What is really useful about them, is that they map circlines (circles or lines) to circlines. As a result, I managed to derive a set of criteria on the $n$ radii for when they can form a Steiner chain. In addition, if such a chain exists, I gave a method how to construct it.
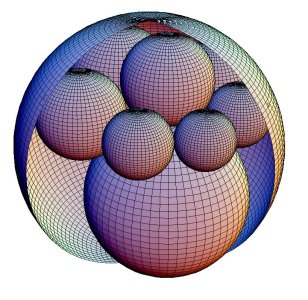
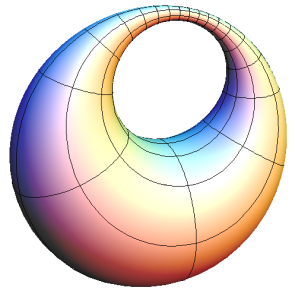
There is a number of generalisations, of which the Soddy's hexlet, which can be thought of as a 3D analogue of a Steiner chain, is a beautiful example and is such that the envelope of the touching spheres is the Dupin cyclide, an inversion of the torus."
The three rotating figures above show: a simple closed Steiner chain with 7 circles, Soddy’s Hexlet and Dupin Cyclide.