Have you ever picked up a glass to find that the coaster it was resting on remains stuck to the bottom? If so, then you have experienced the ability of fluid to stick two surfaces together. When the bottom of the glass is wetted, for example by accidentally spilling a drink, then this fluid can fill the gap between the glass and coaster. The surface tension of the liquid then provides a pulling force on the coaster that keeps it attached to the glass.
It is believed that the climbing ability of insects is aided by this effect, albeit at a smaller scale. Insects are able to walk up walls and across ceilings, and have been observed holding over 100 times their own body weight while remaining adhered. Looking closely beneath their feet, an oily fluid is found that is believed to help stick their feet to a substrate. It has also been noted that the footpads of some insects are soft and deformable.
Inspired by these observations of insects, Oxford Mathematicians Matthew Butler and Dominic Vella have developed a mathematical model to investigate the combination of deformability and adhesion. They consider a system where the footpad is a thin, deformable sheet under tension (like a drum skin) in which the deformability can be varied by changing the tension in the sheet, and the separation of the solid surfaces can be controlled.
Calculations of the equilibria reveal two distinct stable states, distinguished by whether the deformable sheet is in contact with the substrate or not. In both stable states, the adhesion force is found to be always larger than an equivalent rigid case, suggesting that deformation can be good—the surface tension deforms the sheet, increasing the force provided by surface tension: a positive feedback. Further, when the sheet is in contact with the substrate, the adhering force can be orders of magnitude larger than the non-contacting state.
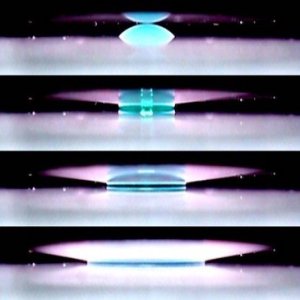
Experiments performed by Finn Box and Thomas Robert show a good qualitative agreement with the theory, as well as demonstrating that adhesion is possible in a lab scale model. However, it was found that adhesion was only maintained if the initial attachment phase took a sufficient length of time. Further study of the dynamical theory suggests this bottleneck is likely to be due to the formation of a fluid dimple as the deformable sheet tries to contact the base, but is resisted by fluid viscosity.
Despite this caveat, the significant increase in adhesion compared to the rigid case means that deformable capillary adhesives are a fruitful area for further investigation. Of particular interest with this system, is that being able to set the tension and separation may allow for high adhesion, whilst also giving easy control of detachment when required. It is hoped that further investigation of similar devices that exploit deformability may lead to advancements in the world of adhesion science.