Wout Moltmaker - Seifert Surface

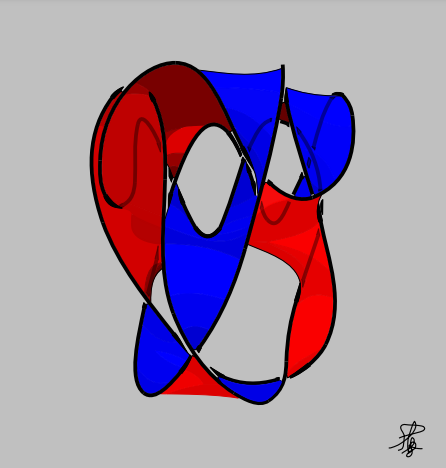
I've hand-drawn a vector image of a Seifert surface: a surface whose boundary is a (mathematical) knot. The knot in the drawing is the 100th prime knot with ten crossings. Seifert surfaces are used to define the genus of a knot. Allowing such surfaces to lie in 4 dimensions gives the related concepts of the topological/smooth slice genus of a knot. A knot that has topological genus 0 but non-zero smooth genus can be used to construct an exotic structure on R4, which is one of the result that earned Michael Freedman his Field's medal.

Wout Moltmaker is a postgraduate student at St Peter's College.