Season's greetings from Oxford Mathematics
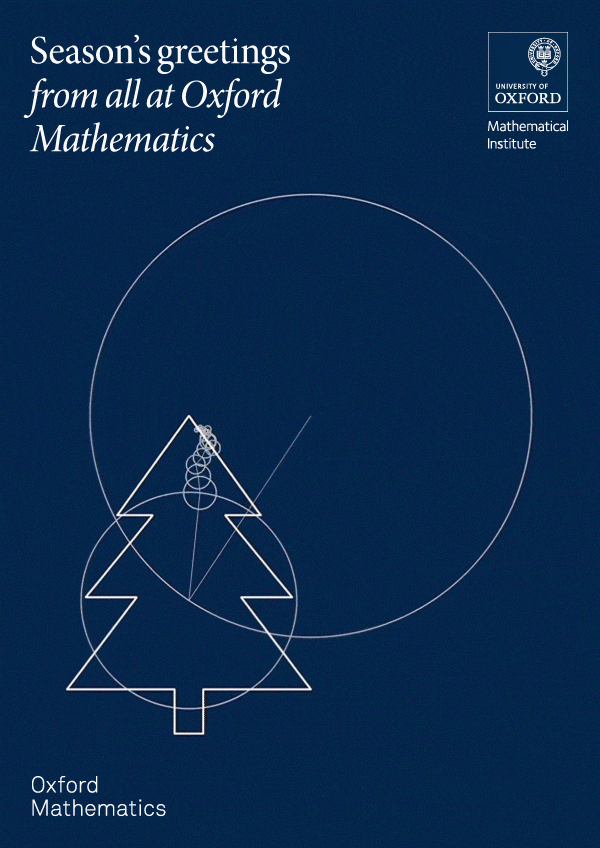
The Oxford Mathematics Christmas tree is drawn using Fourier transform and corresponding epicycles (circles rotating around one another by some vector). Fourier transform allows us to represent a drawing by breaking it up into a sum of 3D spiral sine waves. These are a sum of complex harmonic sinusoids of different frequencies, phases and amplitudes. Following Euler’s formula, if we physically look at this 3D plot from the front, or similarly, mathematically compose it in its polar form, we observe the rotating circles (epicycles).
Fun fact: Fourier transform is widely used for image processing in printing; for example, in image compression and decomposing images into their sine and cosine components (much like how JPEG files work). Printed images are usually composed of dots, named halftone dots, mostly created via Fourier transform.
Here Oxford Mathematician Marina Simonian explains her design. With thanks to our designers at William Joseph for bringing it to life.

