J is for Juggling
The Author
Find out more
Unlocking the mathematical formula of juggling features mathematician Colin Wright, who helped invent siteswap, on More or Less.
The Wikipedia article on siteswap is an excellent place if you want to learn more about the mathematics of juggling (including links to simulators if you want to try to develop your own!).
As juggling is an active motion, some readers may prefer to look at videos of juggling - Colin Wright has an excellent YouTube series on Juggling and Maths.
And if you're interested in what other areas of maths juggling connects to, Juggling, maths and a beautiful mind is an article on Plus exploring just that.
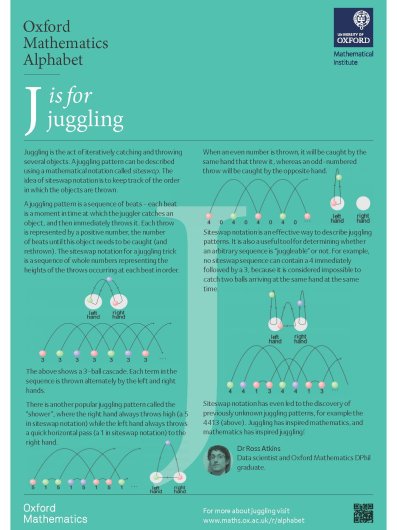
J is for Juggling
Juggling is the act of iteratively catching and throwing several objects. A juggling pattern can be described using a mathematical notation called siteswap. The idea of siteswap notation is to keep track of the order in which the objects are thrown. The notation does not indicate what kind of objects are being juggled (e.g. balls, rings, clubs, etc) or whether a special kind of throw is performed (e.g. under-the-leg or behind-the-back).
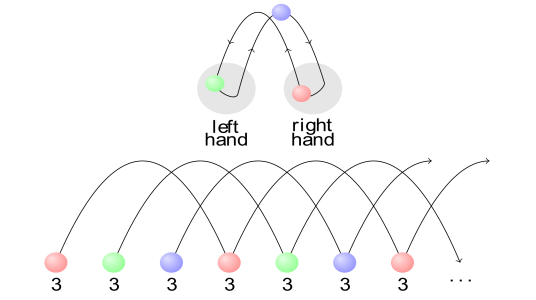
A juggling pattern is a sequence of beats - each beat is a moment in time at which the juggler catches an object, and then immediately throws it. Each throw is represented by a positive integer, equal to the number of beats the object is in the air (so larger numbers indicate higher throws). The siteswap notation for a juggling trick is a sequence of these integers, one for each throw in order. For example, the 3-ball cascade in siteswap notation is simply
Each term in the sequence is thrown alternately by the left and right hands.
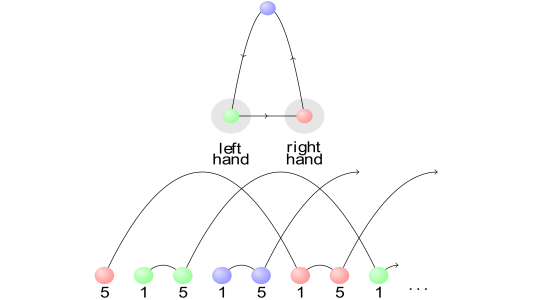
There is another popular juggling pattern called the “shower”, where the right hand always throws high (a 5 in siteswap notation) while the left hand always throws a quick horizontal pass (a 1 in siteswap notation) to the right hand. The shower in siteswap notation is therefore
When an even number is thrown, it will be caught by the same hand that threw it, whereas an odd-numbered throw will be caught by the opposite hand. Therefore the act of juggling two balls in one hand in siteswap notation is
Siteswap notation is an effective way to describe juggling patterns. It is also a useful tool for determining whether an arbitrary sequence is “juggleable” or not. For example, no siteswap sequence can contain a 4 immediately followed by a 3, because it is considered impossible to catch two balls arriving at the same hand at the same time. Siteswap notation has even led to the discovery of previously unknown juggling patterns, for example the 4413. Juggling has inspired mathematics, and mathematics has inspired juggling!