Season 2 Episode 3
Do you like prime numbers? Do you like complex numbers? We've got both! In this episode, Ittihad explains the theory behind Gaussian Primes, which is a theory of prime numbers which are also the complex numbers. We also prove a theorem about writing real primes as the sum of two squares.
Further Reading
Gaussian primes
Ittihad based parts of the presentation on lecture notes by Prof. Keith Conrad at the University of Connecticut here. See this page for more of Prof. Conrad's lecture notes.
Here’s the map of Gaussian primes again, this time in the OOMC colours. Gaussian primes are in blue, and Gaussian integers that are not Gaussian primes are in pink.
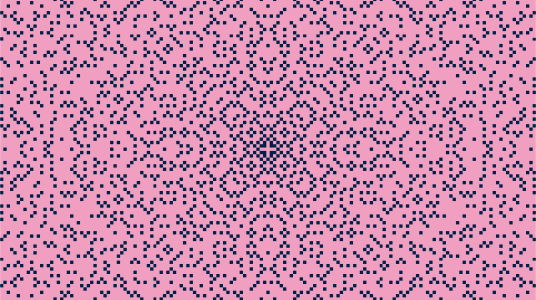
There’s an unsolved problem about the distribution of Gaussian primes above. It goes as follows; if you think of the Gaussian primes as stepping stones, and you’re only allowed to jump from one stepping stone to another one if it’s nearby, can you escape out infinitely far away from the origin? We currently know that if you can only take steps of size 6, then you can’t escape- there’s a wide “moat” around the origin (very far away) that you can’t cross with steps of size 6. No-one knows if there’s a similar moat further out that you can’t cross with steps of size 7. See https://en.wikipedia.org/wiki/Gaussian_moat for more!
Which primes are the sum of two squares?
On the livestream, we showed that if p is a prime of the form 4k+1, then it can be written as a^+b^2. Let’s think about primes of the form 4k+3 (that’s all the other primes in the world, except the number 2). Could any of those primes of the form p=4k+3 be written as a^2+b^2? The answer is “no” and I’ll let you work out for yourself why this is. [Hint: odd/even numbers.]
It’s sometimes helpful to go back to a proof and work out where it breaks down if we change a condition at the start. Here, if we try to copy out the proof from the livestream for a prime of the form 4k+3, then things go wrong when we introduce all those minus signs in the middle of the proof of the Lemma. Sometimes doing little exercises like this is a good way for me to check that I understand the proof.
There is a famous number theory book called “Primes of the form $x^2+ny^2$ by David A. Cox. It’s a very good name for a book! The case $n=1$ is what we’ve been talking about here, and it’s proved by page 12 of Prof Cox’s book. There are then about 350 more pages in the book to cover all other cases for $n>1$!
I should stress here that I’m not encouraging you to read David A Cox’s book (yet!). There is lots of university-level number theory that you need to be familiar with before you can follow modern work in this branch of mathematics. Perhaps the journey we made on the livestream through the complex numbers demonstrates that the mathematical technology used to prove a fact might be much more “advanced” than you would guess from the statement of the fact.
Primes of the form $n^2\pm1$
Here are two very similar questions. One is much much harder than the other.
- Are there infinitely many primes of the form $n^2-1$ where $n$ is an integer?
- Are there infinitely many primes of the form $n^2+1$ where $n$ is an integer?
The answer to the first question is “no” (have a go at proving this yourself!). Nobody knows the answer to the second question.
Here’s a list of some primes of the form $n^2+1$ on the Online Encyclopedia of Integer Sequences. Apart from a couple of small primes, they all end in 1 or 7. Can you work out why? [Hint: Season 1 Episode 0]
Curve sketching
$$y=\frac{x}{\ln(x)}$$
This is a good approximation to the number of (real) primes between 1 and $x$. Yes, it is the reciprocal of a curve sketching question that we’ve had before.
If you want to get in touch with us about any of the mathematics in the video or the further reading, feel free to email us on oomc [at] maths.ox.ac.uk.

