University of Manchester

I will discuss several interesting examples of classes of structures for which there is a sensible first-order theory of "almost all" structures in the class, for certain notions of "almost all". These examples include the classical theory of almost all finite graphs due to Glebskij-Kogan-Liogon'kij-Talanov and Fagin (and many more examples from finite model theory), as well as more recent examples from the model theory of infinite fields: the theory of almost all algebraic extensions and the universal/existential theory of almost all completions of a global field (both joint work with Arno Fehm). Interestingly, such asymptotic theories are sometimes quite well-behaved even when the base theories are not.

The category PAb of profinite abelian groups is an abelian category with many nice properties, which allows us to do most of standard homological algebra. The category PAb naturally embeds into the category TAb of topological abelian groups, but TAb is not abelian, nor does it have a satisfactory theory of tensor products. On the other hand, PAb also naturally embeds into the category CondAb of "condensed abelian groups", which is an abelian category with nice properties. We will show that the embedding of profinite modules into condensed modules (actually, into "solid modules") preserves usual homological notions such Ext and Tor, so that the condensed world might be a better place to study profinite modules than the topological world.

It is well known that the theory of differential-difference fields in characteristic zero has a model companion. Here by a differential-difference field I mean a field with a differential and a difference structure where the operators commute (in other words the difference structure is a differential-endomorphism). The theory DCFA_0 was studied in a series of papers by Bustamante. In this talk I will address the case of positive characteristic.

Deflation is a technique to remove a solution to a problem so that other solutions to this problem can subsequently be found. The most prominent instance is deflation we see in eigenvalue solvers, but recent interest has been in deflation of rootfinding problems from nonlinear PDEs with many isolated solutions (spearheaded by Farrell and collaborators). 

In this talk I’ll show you recent results on deflation techniques for optimisation algorithms with many local minima, focusing on the Gauss—Newton algorithm for nonlinear least squares problems.  I will demonstrate advantages of these techniques instead of the more obvious approach of applying deflated Newton’s method to the first order optimality conditions and present some proofs that these algorithms will avoid the deflated solutions. Along the way we will see an interesting generalisation of Woodbury’s formula to least squares problems, something that should be more well known in Numerical Linear Algebra (joint work with Güttel, Nakatsukasa and Bloor Riley).

Main preprint: https://arxiv.org/abs/2409.14438. 

WoodburyLS preprint: https://arxiv.org/abs/2406.15120

This study introduces a novel suite of historical large language models (LLMs) pre-trained specifically for accounting and finance, utilising a diverse set of major textual resources. The models are unique in that they are year-specific, spanning from 2007 to 2023, effectively eliminating look-ahead bias, a limitation present in other LLMs. Empirical analysis reveals that, in trading, these specialised models outperform much larger models, including the state-of-the-art LLaMA 1, 2, and 3, which are approximately 50 times their size. The findings are further validated through a range of robustness checks, confirming the superior performance of these LLMs.

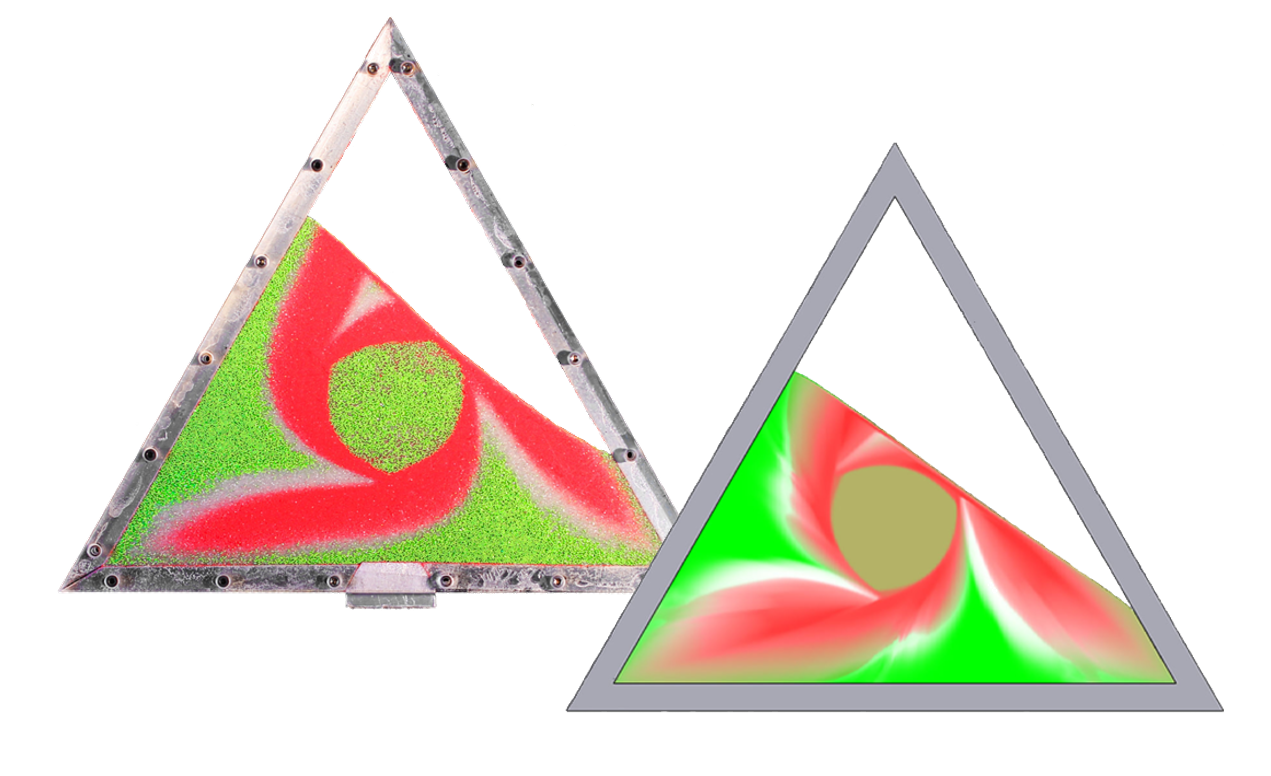
During the last fifteen years, there has been a paradigm shift in the continuum modelling of granular materials; most notably with the development of rheological models, such as the μ(I)-rheology (where μ is the friction and I is the inertial number), but also with significant advances in theories for particle segregation. This talk details theoretical and numerical frameworks (based on OpenFOAM®) which unify these disconnected endeavours. Coupling the segregation with the flow, and vice versa, is not only vital for a complete theory of granular materials, but is also beneficial for developing numerical methods to handle evolving free surfaces. This general approach is based on the partially regularized incompressible μ(I)-rheology, which is coupled to a theory for gravity/shear-driven segregation (Gray & Ancey, J. Fluid Mech., vol. 678, 2011, pp. 353–588). These advection–diffusion–segregation equations describe the evolving concentrations of the constituents, which then couple back to the variable viscosity in the incompressible Navier–Stokes equations. A novel feature of this approach is that any number of differently sized phases may be included, which may have disparate frictional properties. The model is used to simulate the complex particle-size segregation patterns that form in a partially filled triangular rotating drum. There are many other applications of the theory to industrial granular flows, which are the second most common material used after fluids. The same processes also occur in geophysical flows, such as snow avalanches, debris flows and dense pyroclastic flows. Depth-averaged models, that go beyond the μ(I)-rheology, will also be derived to capture spontaneous self-channelization and levee formation, as well as complex segregation-induced flow fingering effects, which enhance the run-out distance of these hazardous flows.

Suppose 𝐺_𝕜 is a connected reductive algebraic 𝕜-group where 𝕜 is an algebraically closed field. If 𝑉_𝕜 is a 𝐺_𝕜-module then, using geometric invariant theory, Kempf has defined the nullcone 𝒩(𝑉_𝕜) of 𝑉_𝕜. For the Lie algebra 𝔤_𝕜 = Lie(𝐺_𝕜), viewed as a 𝐺_𝕜-module via the adjoint action, we have 𝒩(𝔤_𝕜) is precisely the set of nilpotent elements.

We may assume that our group 𝐺_𝕜 = 𝐺 ×_ℤ 𝕜 is obtained by base-change from a suitable ℤ-form 𝐺. Suppose 𝑉 is 𝔤 = Lie(G) or its dual 𝔤* = Hom(𝔤, ℤ) which are both modules for 𝐺, that are free of finite rank as ℤ-modules. Then 𝑉 ⨂_ℤ 𝕜, as a module for 𝐺_𝕜, is 𝔤_𝕜 or 𝔤_𝕜* respectively.

It is known that each 𝐺_ℂ -orbit 𝒪 ⊆ 𝒩(𝑉_ℂ) contains a representative ξ ∈ 𝑉 in the ℤ-form. Reducing ξ one gets an element ξ_𝕜 ∈ 𝑉_𝕜 for any algebraically closed 𝕜. In this talk, we will explain two ways in which we might want ξ to have “good reduction” and how one can find elements with these properties. We will also discuss the relationship to Lusztig’s special orbits.

This is on-going joint work with Adam Thomas (Warwick).

Habegger showed that a subvariety of a fibre power of the Legendre family of elliptic curves is special if and only if it contains a Zariski-dense set of special points. I'll discuss joint work with Gal Binyamini, Harry Schmidt, and Margaret Thomas in which we use pfaffian methods to obtain an effective uniform version of Manin-Mumford for products of CM elliptic curves. Using this we then prove an effective version of Habegger's result.