MAT syllabus practice solutions

updated July 2020 (first version October 2019)

These questions have since been expanded and updated to form the revision material for the MAT livestream.

These are the solutions to the MAT syllabus practice questions.

Solve $x^2-x-1=0$
The quadratic formula gives $x=\frac{1\pm\sqrt{5}}{2}$.
Solve $x^4-x^2-1=0$
Write $y=x^2$ to get a quadratic for $y$. This is the quadratic above for $y$, so $x^2=\frac{1\pm\sqrt{5}}{2}$. But $x^2\geq0$ so $x=\pm\sqrt{\frac{1+\sqrt{5}}{2}}$.
Write $x^2+4x+3$ in the form $(x+a)^2+b$
$(x+2)^2-1$
How many real solutions does $x^2+bx+1=0$ have? Find the different cases in terms of $b$.
The discriminant, $b^2-4$, is positive if $b>2$ or $b<-2$, negative if $-2<b<2$ and zero if $b=\pm2$. So there are two real solutions if $b>2$ or if $b<-2$, one real solution if $b=\pm2$ and no real solutions otherwise.
Factorise $x^2+4x+3$
$(x+1)(x+3)$
Let $p(x)=x^3-13x^2-65x-51$. Check that $p(17)=0$. Factorise $p(x)$.
$p(17)=17^3-13\times17^2-65\times17-51=17\left(17^2-13\times 17-65-3\right)=17^2\left(17-13-4\right)=0$. So $(x-17)$ is a factor. Polynomial division gives $p(x)=(x-17)(x^2+4x+3)$, so $p(x)=(x-17)(x+1)(x+3)$.

Solve the simultaneous equations $x+y=1$ and $x-y=3$.
$x=2$ and $y=-1$.
For which values of $x$ is it true that $x^2+4x+3>0$?
$x>-1$ or $x<-3$.
Expand $(2x+3)^3$
$8x^3+36 x^2+54x+27$
I've got four playing cards; the ace and king of clubs, and the ace and king of hearts. I shuffle the cards together and deal them out left to right. What's the probability that the kings and aces alternate? (they alternate if they are either arranged as $AKAK$ or $KAKA$)
There are 24 possible orders for the cards. Eight of these have alternating kings and aces, so the probability is 1/3.

Differentiate $x^{17}$ with respect to $x$.
$17x^{16}$
Differentiate $\sqrt{x}$ with respect to $x$.
$\frac{1}{2\sqrt{x}}$
Differentiate $e^{3x}$ with respect to $x$.
$3e^{3x}$
Differentiate $2e^{-x}-x^2$ with respect to $x$.
$-2e^{-x}-2x$
Find the tangent to the curve $y=e^x+1$ at $x=2$.
$y=e^2(x-2)+e^2+1$
Find the normal to the parabola $y=x^2$ at $x=3$.
$y=-\frac{1}{6}(x-3)+9$
Find the turning points of the curve $y=x^4-2x^3+x^2$. Identify whether the turning points are maxima or minima.
Turning points at $x=0$ (minimum), $x=\frac{1}{2}$ (maximum), $x=1$ (minimum).
For which values of $x$ is $y=x^4-2x^3+x^2$ increasing? For which values of $x$ is it decreasing?
Increasing for $0<x<\frac{1}{2}$ and for $1<x$. Decreasing for $x<0$ and for $\frac{1}{2}<x<1$.
Two points $A$ and $B$ are on the curve $y=x^3+x^2+x+1$. $A$ is held fixed at $(1,4)$. The point $B$ is moved along the curve towards $A$. What happens to the line through $A$ and $B$?
The tangent at $A$ is $y=6x-2$. If the line $AB$ has equation $y=mx+c$ say, then $m$ gets closer and closer to 6 and $c$ gets closer and closer to $-2$.

Suppose that the derivative of a polynomial $p(x)$ with respect to $x$ is $q(x)$. Find $\displaystyle \int q(x)\,\mathrm{d}x$.
$p(x)+c$ where $c$ is a constant
Find the area enclosed by the polynomial $x^2+4x+3=0$ and the $x$-axis.
$\frac{4}{3}$
Find $\displaystyle \int_{-1}^1 1+x+x^2+x^3+x^4+x^5+x^6\,\mathrm{d}x$
Note that $\int_{-1}^1 x^a \,\mathrm{d}x=0$ for $a$ odd. The integral is 2$\left(1+\frac{1}{3}+\frac{1}{5}+\frac{1}{7}\right)=\frac{352}{105}$.

Sketch graphs of
$$y=x^2+4x+3,\quad y=x^3+4x^2+3x,\quad y=2^x, \quad y=\log_2 x$$ on separate axes.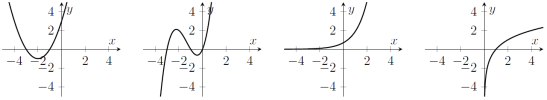
Sketch graphs of $y=\sin x$, $y=\cos x$ and $y=\tan x$ on the same axes.

Let $f(x)=x^2+4x+3$. If you didn't sketch a graph of this before, sketch one now.
Sketch a graph of $y=f(x+2)$.
Sketch a graph of $y=3 f( 2 x)$.

Add the vectors $\displaystyle \left(\begin{matrix}1\\2\end{matrix}\right)$ and $\displaystyle \left(\begin{matrix}3\\-2\end{matrix}\right)$.
$\displaystyle \left(\begin{matrix}
4\\0
\end{matrix}\right)$
Find the equation of the line through $(1,0)$ and $(0,-1)$.
$y=x-1$
Find the equation of the line through $(1,2)$ with gradient 3.
$y=3(x-1)+2=3x-1$
A circle has centre $(-1,4)$ and radius 3. Write down an equation for the circle.
$(x+1)^2+(y-4)^2=9$
What's the area of this circle?
$9\pi$
Points $A$ and $B$ lie on a circle with centre $O$ and radius 1. The angle $\angle AOB$ is 120$^\circ$. Find the length of the arc between $A$ and $B$. Find the area enclosed by that arc and the radii $OA$ and $OB$.
It's a third of a circle, so the arc length is $2\pi/3$ and the area is $\pi/3$.

Solve $\sin x = \frac{1}{2}$.
$x=30^\circ+n\times 360^\circ$, or $x=150^\circ+n\times 360^\circ$, for any whole number $n$.
Solve $\tan x = 1$.
$x=45^\circ+n\times 180^\circ$ for any whole number $n$
Write $\cos^4x+\cos^2x$ in terms of $\sin x$.
$(1-\sin^2x)^2+(1-\sin^2 x)=2-3\sin^2x+\sin^4x$.
Simplify $\cos(450^{\circ} -x)$
$\sin x$
A triangle $ABC$ has side lengths $AB=3$ and $BC=2$, and the angle $\angle ABC=120^\circ$. Find the remaining side length $AC$, the area of the triangle, and an expression for $\sin \angle BCA$.
Cosine rule; $AC=\sqrt{19}$. The area of the triangle is $3\sqrt{3}/2$. Sine rule; $\sin \angle BCA=(3\sqrt{3}/2\sqrt{19})$

A sequence is defined by $a_0=1$, $a_1=1$, $a_2=1$, and
$$a_n=a_{n-1}+a_{n-2}+a_{n-3}\quad\mbox{for $n\geq 3$.}$$
Find $a_{10}$.
$a_{3}=3$, $a_4=5$, $a_5=9$, $a_6=17$, $a_7=31$, $a_8=57$, $a_9=105$, $a_{10}=193$.
A sequence has first term 3 and each subsequent term is 5 more than the previous term. Find the sum of the first four terms.
$4\times 3+\frac{4\times 3}{2}\times 5=42$
A sequence has first term 4 and each subsequent term is 6 times more than the previous term. Find the sum of the first four terms.
$4\left(1+6+6^2+6^3\right)=4\frac{6^4-1}{6-1}=4\frac{1295}{5}=4\times 259=1036$.
When does the sum $1+x^3+x^6+x^9+x^{12}+...$ converge? Simplify it in the case that it converges.
Converges when $-1<x<1$. In that case, it converges to $1/(1-x^3)$.

For more MAT resources, see the MAT page.