C is for Calabi-Yau Manifolds
The Author

Dr Andreas Braun
Postdoctoral Research Assistant
Andreas Braun is a researcher working in the mathematical physics group at the Mathematical Institute. He is working on dualities of string and field theories, as well as compactifications of string theory. This involves a whole lot of fascinating mathematics, in particular (algebraic) geometry. He initially studied as a theoretical particle physicist, becoming involved in string theory and then needing mathematics to make further progress.
Find out more
Further reading about Calabi-Yau manifolds (from popular science to research monograph):
- The Elegant Universe by Brian Greene
- The Shape of Inner Space by Shing-Tung Yau and Steve Nadis
- The scholarpedia article on Calabi-Yau manifolds (written by Shing-Tung Yau)
- Mirror Symmetry by Hori et al.
- Mirror Symmetry and Algebraic Geometry by Cox and Katz
- Calabi-Yau Manifolds and Related Geometries by Gross, Huybrechts and Joyce
We have a whole online gallery of our models of geometric surfaces(including Kummer surfaces).
Hidden Dimensions explains more about the intertwining of physics and geometry.
String Theory, Duality and Art: how the Higgs boson and Turner Prize collide investigates the connection between physics, art, and mathematics.
C is for Calabi-Yau Manifolds
Calabi-Yau manifolds have become a topic of study in both mathematics and physics, dissolving the boundaries between the two subjects.
A manifold is a type of geometrical space where each small region looks like normal Euclidean space. For example, an ant on the surface of the Earth sees its world as flat, rather than the curved surface of the sphere. Calabi-Yau manifolds are complex manifolds, that is, they can be disassembled into patches which look like flat complex space. What makes them so special is that these patches can only be joined together by the complex analogue of a rotation. The only particularly nice (compact) solution to this condition in one complex dimension (=2 real dimensions) is a torus, but things get much more interesting in higher dimensions. While it is hard to picture any manifold with more than 2 real dimensions, it is not so hard to construct examples by means of algebraic equations.
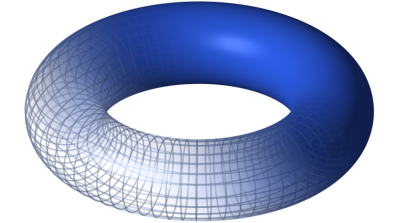
We can describe an n-dimensional sphere by considering the (real) solutions of
$$x_1^2 + \dotsc + x_{n+1}^2 = r^2.$$
Likewise an example of a complex three-dimensional Calabi-Yau manifold is given by the (complex) solutions to
$$x_1^5 + x_2^5 + x_3^5 + x_4^5 + \psi x_1x_2x_3x_4 = 1$$
for a parameter $\psi$. This famous Calabi-Yau manifold is called the 'quintic'.

Proving a conjecture of Eugenio Calabi, Shing-Tung Yau has shown that Calabi-Yau manifolds have a property which is very interesting to physics. Einstein's equations show that spacetime curves according to the distribution of energy and momentum. But what if space is all empty? By Yau's theorem, not only is flat space a solution but so are Calabi-Yau manifolds.
For this reason, Calabi-Yau spaces are possible candidates for the shape of extra spatial dimensions in String Theory. Here, one is particularly interested in (complex) three-dimensional Calabi-Yau manifolds such as the quintic, known as threefolds. Every example of a Calabi-Yau threefold then gives rise to a different universe with different sets of elementary particles and particle interactions. This makes the question of classification, that is, which Calabi-Yau threefolds there are and what their properties are, tremendously interesting. However, to date it is not even clear whether there are finitely many. So far, physicists and mathematicians have constructed roughly half a billion examples.
When constructing examples of Calabi-Yau manifolds, physicists made a strange observation: they naturally seem to occur in pairs where every Calabi-Yau manifold can be associated with a different partner Calabi-Yau manifold, its 'mirror'. By a slight tweak of string theory, one can swap a Calabi-Yau manifold with its mirror without changing physics at all. This has led physicists to propose many correspondences between the geometry of Calabi-Yau manifolds and their mirrors which (used to) seem proposterous to mathematicians. Most famously, there are formulas, first derived by Oxford mathematicians Candelas, de la Ossa and collaborators, counting in how many different ways any curve can be embedded into a Calabi-Yau manifold. This is a hard problem in mathematics! Amazingly, many 'stringy' theorems have been subsequently proven rigorously and have inspired a lot of research in mathematics.
This is a projection of a (complex) two-dimensional Calabi-Yau manifold, also called a K3 surface. The model is part of the Mathematical Institute's collection, and is currently on display in the Mezzanine of the Andrew Wiles Building.



