Polynomials Solutions
Part of the Oxford MAT Livestream.
Revision Questions
- \(p(2)=2\times 2^3-5\times 2^2+7\times 2-3=7.\) So \(p(2)=7\).
- We can rearrange to \(x^2-x-1=0\) and then use the quadratic formula for \(x=\frac{1}{2}\left(1\pm \sqrt{5}\right)\). If we choose the solution with the \(+\) sign then we'll get a positive number.
- In this case, the discriminant "\(b^2-4ac\)" is \(5^2-4\times 2 \times 1=17\).
- The discriminant for this quadratic is \(1-4k\). There are exactly two real solutions if this is positive, which happens when \(k<\frac{1}{4}\).
- This is not a quadratic, but if we change variable by writing \(u=x^2\) then we get \(u^2-u+k=0\). That's got two real solutions if \(k<\frac{1}{4}\), one real solution if \(k=\frac{1}{4}\), and no real solutions if \(k>\frac{1}{4}\) (thinking about the discriminant again). But let's be careful, because that's the number of solutions there are for \(u\), and we really want to know how many solutions there are for \(x\).
If there are no real solutions for \(u\) then there can't be any real solutions for \(x\). So that rules out \(k>\frac{1}{4}\). If there's exactly one solution for \(u\) then we might get two real solutions for \(x\); they'd be \(\pm\sqrt{u}\), but that only works if the solution for \(u\) is a positive number. In the case \(k=\frac{1}{4}\), we've got one solution for \(u\), and if we write down the quadratic formula then that solution is actually \(\frac{1}{2}\), so we do get two real solutions for \(x\). In the other remaining case \(k<\frac{1}{4}\) there are two real solutions for \(u\). That could give us as many as four real solutions for \(x\). We'd get exactly two real solutions for \(x\) if and only if one of the solutions for \(u\) is positive and one is negative. Thinking about the factorisation \((u-a)(u-b)\), we can see that the constant term \(k\) in our quadratic for \(u\) would have to be negative for there to be one positive solution and one negative solution. So we would get two real solutions for \(x\) only if \(k<0\).
Putting all that together, there are two real solutions for \(x\) if \(k<0\) or if \(k=\frac{1}{4}\), and for no other values of \(k\). - The discriminant is \(b^2-4\). That's positive (and the quadratic has two real solutions) if \(b>2\) or if \(b<-2\). If \(b=\pm 2\) then the quadratic has one solution. If \(-2<b<2\) then the quadratic has no real solutions.
- If I imagine multiplying out \((x+a)^2\), then I would get a term \(2ax\), and I want that to match with the \(4x\) term. So I'll take \(a=2\). Then if I multiply out \((x+2)^2\), I'd get a term \(+4\) at the end; that's not quite what I want, so I'll take \(b=-1\) to fix the constant coefficient of this quadratic. I get \((x+2)^2-1\).
- We can write this polynomial as \(-2(x-2)^2+13\). The extreme value is therefore 13. This is a maximum because \(-2(x-2)^2\leq 0\).
- First we're asked to check that \(17^3-13\times 17^2-65\times 17-51=0\). To make this easier, don't work out the terms individually. Instead pull out factors of \(17\);
\(17^3-13\times 17^2-65\times 17-51=17\left(17^2-13\times 17 -65-3\right)\) because \(51=3\times 17\).
\(17^2-13\times 17 -68=17\left(17-13-4\right)\) because \(68=4\times 17\).
\(17-13-4=0\) so each line above is equal to zero. - By the Factor Theorem, if \(p(17)=0\) then \((x-17)\) is a factor of the polynomial. Doing some polynomial division, we can work out that \(p(x)=(x-17)(x^2+4x+3)\). We can then write \(x^2+4x+3=\left(x+3\right)\left(x+1\right)\) and we've factorised \(p(x)\).
- The polynomial \(p(x)\) has a factor of \((x-2)\).
- We have $p(x)=(x-2)q(x)$ for some polynomial $q(x)$, so $p(2)=(2-2)q(2)=0$.
- Check that \(f(2)=0\).
Now factorise \(f(x)=(x-2)(x^3-4x^2+5x-2)\). Look for more roots; perhaps \(x=2\) is a repeated root? In fact $2^3-4\times 2^2+5\times 2-2=0$ so it is a repeated root.
\(f(x)=(x-2)^2(x^2-2x+1)\) and we can recognise that quadratic as \((x-1)^2\).
So \(f(x)=(x-1)^2(x-2)^2\). - We might notice that \(p(1)=0\). Then write \(p(x)=(x-1)(x^2-5x+6)\) and factorise the quadratic for \(p(x)=(x-1)(x-2)(x-3)\).
- \(p(3)=-9\) is not zero, so \((x-3)\) is not a factor.
- Yes, the polynomial could have a repeated root. For example, \(p(x)=2(x-1)^2(x-2)\)
- \(y=2x^6+x^3+1\). Choosing \(u=x^3\) gives \(y=2u^3+u+1\).
- \(y=x+\sqrt{2x}\). Choosing \(u=\sqrt{x}\) gives \(y=u^2+\sqrt{2}u\).
- \(y=3e^{-3x}+6e^{-6x}\). Choosing \(u=e^{-3x}\) gives \(y=3u+6u^2\).
- \(\displaystyle y=\frac{1+x}{(1-x)^2}\). We can rearrange this to \(\displaystyle y=\frac{(x-1)+2}{(1-x)^2}=\frac{-1}{1-x}+\frac{2}{(1-x)^2}\). Choosing \(\displaystyle u=\frac{1}{1-x}\) gives \(y=-u+2u^2\).
- \(q(x)\) could be \(17(x-2)(x+3)(x-1)\) or \(39(x-2)^2(x+3)^2(x-1)^2\) or \(-(x-3)(x-2)(x-1)x(x+1)(x+2)(x+3)\). We aren't told if these are repeated roots or not, or whether there are any other roots, or what the leading coefficient is.
- \(v(1)=3+a+b\) and that must be zero. Try polynomial division; $$v(x)=(x-1)(x^2+3x+(a+3)),$$ provided that $3+a+b=0$. Now we want \(x=1\) to be root of that quadratic, so we need \(1+3+a+3=0\). Solve these equations for $a=-7$ and $b=4$.
MAT Questions
MAT 2016 Q1F
- $(x+1)$ is a factor of this polynomial if and only if $-1$ is a root of the polynomial, which would mean that $$(3+(-1)^2)^n-(-1+3)^n(-1-1)^n=0.$$
- This simplifies to $$4^n-2^n(-2)^n=0.$$
- If $n$ is even then $(-2)^n$ is the same as $2^n$ and the equality is true. If $n$ is odd then we have $4^n+4^n$ on the left-hand side, which is not zero for any positive integer $n$.
- So the answer is (b).
MAT 2015 Q1I
Here's a sketch
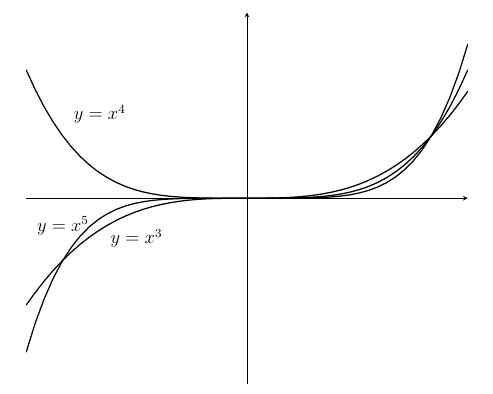
- The three curves all pass through $(0,0)$ and $(1,1)$. The curves $y=x^3$ and $y=x^5$ both pass through $(-1,-1)$.
- There's one region above all three curves, one region below all three curves, and a total of seven regions between the curves.
- The answer is (d).
MAT 2007 Q2
(i) Plugging in $n=3$, we have $f_3(x)=(\left(2+(-2)^3\right))x^2+(3+3)x+3^2=-6x^2+6x+9$.
Completing the square, we can write this as $\displaystyle -6\left(x-\frac{1}{2}\right)^2+\frac{21}{2}$
The polynomial is usually a quadratic (unless the leading coefficient $2+(-2)^n$ happens to be zero), in which case it has a maximum if and only if the leading coefficient is negative (if it's a ``sad" quadratic), which happens if $n$ is odd. Watch out for the special case though; $2+(-2)^n$ is zero if $n=1$, in which case the polynomial is a linear function without a maximum.
(ii) \(f_1(x)=4x+1\).
\(f_1(f_1(x))=4(4x+1)+1=4^2 x+4+1=16x+5\).
\(f_1(f_1(f_1(x)))=4(4^2x+4+1)+1=4^3x+16+4+1=64x+21\)
In general, \(f_1(f_1(\cdots f_1(x)\cdots))\) with \(f_1\) applied $k$ times is equal to \(4^k x+4^{k-1}+4^{k-2}+\dots+4+1\).
The constant term is a geometric series, so we can simplify to $$
4^k x+ \frac{4^k-1}{4-1}=4^k x +\frac{4^k-1}{3}.
$$
(iii) \(f_2(x)=6x^2+5x+4\) is a quadratic. Each time we repeatedly square, the degree gets multiplied by 2. So the degree of \(f_2(f_2(\cdots f_2(x)\cdots))\) with \(f_2\) applied $k$ times is \(2^k\).
Extension
- $f_n(x)$ with $n>2$ is still a quadratic, so the degree of \(f_n(f_n(\cdots f_n(x)\cdots))\) with \(f_n\) applied $k$ times is $2^k$ just like in the last part of the question.
- Let's look at what happens for $f(x)=ax^2$ for real non-zero $a$ (only the highest power really matters for this question). $f(f(x))=a(ax^2)^2=a^3x^4$ and $f(f(f(x)))=a(a^3x^4)^2=a^7x^8$, so it looks like the coefficient of $x^{2^k}$ is $a^{2^k-1}$. For the quadratic we're talking about here, the coefficient ends up being $(2+(-2)^n)^{2^k-1}$.
- For $n$ odd and greater than 3, $g$ is a quadratic with a maximum value. $n=3$ is special; $g_3(x)=9$. That has a maximum value of 9 (which is happens to take for all $x$).
- For $n\neq 3$ the degree is $2^k$ again. For $n=3$ the degree is zero because the outcome after all those function applications is still just the value 9.
MAT 2011 Q2
(i) Multiply both sides by $x$ to get $x^4=2x^2+x$.
Then multiply both sides by $x$ again for $x^5=2x^3+x^2$. Now use the fact that $x^3=2x+1$ to write
$x^5=2(2x+1)+x^2=2+4x+x^2$.
(ii) In general we can multiply by $x$ and use the initial fact about $x^3$ to remove any $x^3$ term we get. In general, it looks like this;
$$
x^{k+1}=\left(x^k\right)x=\left(A_k+B_k x + C_k x^2\right)x=A_k x + B_k x^2 + C_k x^3 = A_k x + B_k x^2 + C_k \left(2x+1\right).
$$
Now remember that $x^{k+1}=A_{k+1}+B_{k+1}x+C_{k+1}x^2$. We can match this up with the expression on the right by taking $A_{k+1}=C_k$ and $B_{k+1}=A_k+2C_k$ and $C_{k+1}=B_k$.
(iii) By the previous part, $$A_{k+1}+C_{k+1}-B_{k+1}=C_k+B_k-(A_k+2C_k).$$
That simplifies to $B_k-A_k-C_k$. So $D_{k+1}=-D_k$.
We can also note that $D_4=1$, so $D_5=-1$ and $D_6=1$ and so on; we have $D_k=(-1)^k$. Then use the definition of $D_k$ and rearrange $A_k+C_k-B_k=(-1)^k$ by adding $B_k$ to both sides.
(iv) We're asked to show that $A_{k+1}+C_{k+1}+A_{k+2}+C_{k+2}=A_{k+3}+C_{k+3}$. The way to approach this which is clearest to me is to use the previous part to replace the $A_{k+3}$ and $C_{k+3}$ for things with subscript $k+2$, then replace everything that has a subscript $k+2$ for things with subscript $k+1$, and then hope that everything balances.
I have $A_{k+1}+C_{k+1}+(A_{k+2}+C_{k+2})=A_{k+1}+C_{k+1}+(C_{k+1}+B_{k+1})$ on the left.
I have $A_{k+3}+(C_{k+3})=C_{k+2}+(B_{k+2})=B_{k+1}+(A_{k+1}+2C_{k+1})$ on the right.
[The brackets here are just to make it clearer which terms I'm replacing with which.]
These are equal, so the fact is true.
Alternatively, replace the $A_k+C_k$ terms with $B_k+(-1)^k$ and go from there.
Extension
- Given $x^2=x+1$, we could multiply both sides by $x$ for $x^3=x^2+x$, then replace the $x^2$ for $x+1$ to get $x^3=2x+1$. The roots of that quadratic are $\displaystyle x=\frac{1\pm \sqrt{5}}{2}$. The other solution to $x^3=2x+1$ is $x=-1$.
The quantity $D_k$ is just the value of the quadratic at $x=-1$, and so we have $$A_k+B_k(-1)+C_k(-1)^2=(-1)^k.$$

