First all-flavor search for transient neutrino emission using 3-years of IceCube DeepCore data
 Abbasi, R Ackermann, M Adams, J Aguilar, J Ahlers, M Ahrens, M Alispach, C Alves, A Amin, N Andeen, K Anderson, T Ansseau, I Anton, G Argüelles, C Axani, S Bai, X V., A Barbano, A Barwick, S Bastian, B Basu, V Baum, V Baur, S Bay, R Beatty, J Becker, K Tjus, J Bellenghi, C BenZvi, S Berley, D Bernardini, E Besson, D Binder, G Bindig, D Blaufuss, E Blot, S Böser, S Botner, O Böttcher, J Bourbeau, E Bourbeau, J Bradascio, F Braun, J Bron, S Brostean-Kaiser, J Burgman, A Busse, R Campana, M Chen, C Chirkin, D Choi, S Clark, B Clark, K Classen, L Coleman, A Collin, G Conrad, J Coppin, P Correa, P Cowen, D Cross, R Dave, P De Clercq, C DeLaunay, J Dembinski, H Deoskar, K De Ridder, S Desai, A Desiati, P de Vries, K de Wasseige, G de With, M DeYoung, T Dharani, S Diaz, A Díaz-Vélez, J Dujmovic, H Dunkman, M DuVernois, M Dvorak, E Ehrhardt, T Eller, P Engel, R Evans, J Evenson, P Fahey, S Fazely, A Fiedlschuster, S Fienberg, A Filimonov, K Finley, C Fischer, L Fox, D Franckowiak, A Friedman, E Fritz, A Fürst, P Gaisser, T Gallagher, J Ganster, E Garrappa, S Gerhardt, L Ghadimi, A Glauch, T Glüsenkamp, T Goldschmidt, A Gonzalez, J Goswami, S Grant, D Grégoire, T Griffith, Z Griswold, S Gündüz, M Haack, C Hallgren, A Halliday, R Halve, L Halzen, F Minh, M Hanson, K Hardin, J Haungs, A Hauser, S Hebecker, D Helbing, K Henningsen, F Hickford, S Hignight, J Hill, C Hill, G Hoffman, K Hoffmann, R Hoinka, T Hokanson-Fasig, B Hoshina, K Huang, F Huber, M Huber, T Hultqvist, K Hünnefeld, M Hussain, R In, S Iovine, N Ishihara, A Jansson, M Japaridze, G Jeong, M Jones, B Joppe, R Kang, D Kang, W Kang, X Kappes, A Kappesser, D Karg, T Karl, M Karle, A Katz, U Kauer, M Kellermann, M Kelley, J Kheirandish, A Kim, J Kin, K Kintscher, T Kiryluk, J Klein, S Koirala, R Kolanoski, H Köpke, L Kopper, C Kopper, S Koskinen, D Koundal, P Kovacevich, M Kowalski, M Krings, K Krückl, G Kurahashi, N Kyriacou, A Gualda, C Lanfranchi, J Larson, M Lauber, F Lazar, J Leonard, K Leszczyńska, A Li, Y Liu, Q Lohfink, E Mariscal, C Lu, L Lucarelli, F Ludwig, A Luszczak, W Lyu, Y Ma, W Madsen, J Mahn, K Makino, Y Mallik, P Mancina, S Mariş, I Maruyama, R Mase, K McNally, F Meagher, K Medici, M Medina, A Meier, M Meighen-Berger, S Merz, J Micallef, J Mockler, D Momenté, G Montaruli, T Moore, R Morse, R Moulai, M Naab, R Nagai, R Naumann, U Necker, J Neer, G Nguyên, L Nielsen, M Niederhausen, H Nisa, M Nowicki, S Nygren, D Pollmann, A Oehler, M Olivas, A O'Sullivan, E Pandya, H Pankova, D Park, N Parker, G Paudel, E Peiffer, P Heros, C Philippen, S Pieloth, D Pieper, S Pizzuto, A Plum, M Popovych, Y Porcelli, A Rodriguez, M Price, P Przybylski, G Raab, C Raissi, A Rameez, M Rawlins, K Rea, I Rehman, A Reimann, R Renschler, M Renzi, G Resconi, E Reusch, S Rhode, W Richman, M Riedel, B Robertson, S Roellinghoff, G Rongen, M Rott, C Ruhe, T Ryckbosch, D Cantu, D Safa, I Herrera, S Sandrock, A Sandroos, J Santander, M Sarkar, S Satalecka, K Scharf, M Schaufel, M Schieler, H Schlunder, P Schmidt, T Schneider, A Schneider, J Schröder, F Schumacher, L Sclafani, S Seckel, D Seunarine, S Shefali, S Silva, M Smithers, B Snihur, R Soedingrekso, J Soldin, D Spiczak, G Spiering, C Stachurska, J Stamatikos, M Stanev, T Stein, R Stettner, J Steuer, A Stezelberger, T Stokstad, R Strotjohann, N Stuttard, T Sullivan, G Taboada, I Tenholt, F Ter-Antonyan, S Tilav, S Tischbein, F Tollefson, K Tomankova, L Tönnis, C Toscano, S Tosi, D Trettin, A Tselengidou, M Tung, C Turcati, A Turcotte, R Turley, C Twagirayezu, J Ty, B Unger, E Elorrieta, M Vandenbroucke, J van Eijk, D van Eijndhoven, N Vannerom, D van Santen, J Verpoest, S Vraeghe, M Walck, C Wallace, A Watson, T Weaver, C Weindl, A Weiss, M Weldert, J Wendt, C Werthebach, J Weyrauch, M Whelan, B Whitehorn, N Wiebe, K Wiebusch, C Williams, D Wolf, M Woschnagg, K Wrede, G Wulff, J Xu, X Xu, Y Yanez, J Yoshida, S Yuan, T Zhang, Z (10 Nov 2020)
Generating ultradense pair beams using 400 GeV/c protons
 Arrowsmith, C Shukla, N Charitonidis, N Boni, R Chen, H Davenne, T Dyson, A Froula, D Gudmundsson, J Huffman, B Kadi, Y Reville, B Richardson, S Sarkar, S Shaw, J Silva, L Simon, P Trines, R Bingham, R Gregori, G Physical Review Research volume 3 (10 May 2021)
Blast from the past: Constraints on the dark sector from the BEBC WA66
beam dump experiment
 Marocco, G Sarkar, S SciPost Physics volume 10 (19 Feb 2021) http://arxiv.org/abs/2011.08153v2
Tue, 24 Nov 2020
14:30
Virtual

“Chiral” field theory, fishnets and integrable spin chains

Stefano Negro
(New York University)
Further Information

Please contact Erik Panzer or Ömer Gürdoğan to be added to the mailing list and receive joining instructions to the online seminar.

Abstract

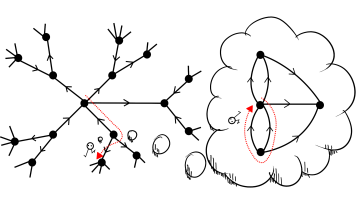
In this talk I will review the work that has been done by me, N. Gromov, V. Kazakov, G. Korchemsky and G. Sizov on the analysis of fishnet Feynman graphs in a particular scaling limit of $\mathcal N=4$ SYM, a theory dubbed $\chi$FT$_4$. After introducing said theory, in which the Feynman graphs take a very simple fishnet form — in the planar limit — I will review how to exploit integrable techniques to compute these graphs and, consequently, extract the anomalous dimensions of a simple class of operators.

Vicky Neale's 'Analysis' Student Lecture now on YouTube

The second in the series of Student Lectures that we are making publicly available this Autumn is from Vicky Neale. Vicky is one of our most popular lecturers and this lecture is from her First Year Analysis course. 

The course introduces students to a rigorous definition of convergence, allowing them to develop their previous understanding of sequences and series and to prove key results about convergence, leading on to subsequent Analysis courses addressing continuity, differentiability and integrability of functions.

Fri, 20 Nov 2020
16:00
Virtual

Polarizations and Symmetries of T[M] theories

Du Pei
(Harvard)
Abstract

I will lead an informal discussion centered on discrete data that need to be specified when reducing 6d relative theories on an internal manifold M and how they determine symmetries of the resulting theory T[M].

Subscribe to