Searches for sterile neutrinos with the IceCube detector
Aartsen, M
Abraham, K
Ackermann, M
Adams, J
Aguilar, J
Ahlers, M
Ahrens, M
Altmann, D
Andeen, K
Anderson, T
Ansseau, I
Anton, G
Archinger, M
Argüelles, C
Arlen, T
Auffenberg, J
Axani, S
Bai, X
Barwick, S
Baum, V
Bay, R
Beatty, J
Becker Tjus, J
Becker, K
BenZvi, S
Berghaus, P
Berley, D
Bernardini, E
Bernhard, A
Besson, D
Binder, G
Bindig, D
Blaufuss, E
Blot, S
Boersma, D
Bohm, C
Börner, M
Bos, F
Bose, D
Böser, S
Botner, O
Braun, J
Brayeur, L
Bretz, H
Burgman, A
Casey, J
Casier, M
Cheung, E
Chirkin, D
Christov, A
Physical Review Letters
volume 117
issue 7
(08 Aug 2016)
Fri, 28 Oct 2016
14:00 -
15:00
L2
Cracking the functions of morphing forms in nature: from plant shoots to the dandelion fruit
Dr Naomi Nakayama
(Molecular Plant Sciences University of Edinburgh)
Fri, 21 Oct 2016
14:00 -
15:00
L3
Computational multiscale modelling of traumatic brain injury
Dr Antoine Jerusalem
(Dept of Engineering Science University of Oxford)
I is for Inverse Problems by Dr Patrick Farrell.
Wed, 19 Oct 2016
15:00
15:00
L5
Cryptanalysis of the Algebraic Eraser
Simon Blackburn
(Royal Holloway University of London)
Abstract
The Algebraic Eraser is a cryptosystem (more precisely, a class of key
agreement schemes) introduced by Anshel, Anshel, Goldfeld and Lemieux
about 10 years ago. There is a concrete instantiation of the Algebraic
Eraser called the Colored Burau Key Agreement Protocol (CBKAP), which
uses a blend of techniques from permutation groups, matrix groups and
braid groups. SecureRF, the company owning the trademark to the
Algebraic Eraser, is marketing this system for lightweight
environments such as RFID tags and other Internet of Things
applications; they have proposed making this scheme the basis for an
ISO RFID standard.
This talk gives an introduction to the Algebraic Eraser, a brief
history of the attacks on this scheme using ideas from group-theoretic
cryptography, and describes the countermeasures that have been
proposed. I would not recommend the scheme for the proposed
applications: the talk ends with a brief sketch of a recent convincing
cryptanalysis of this scheme due to Ben-Zvi, Blackburn and Tsaban
(which appeared at CRYPTO this summer), and significant attacks
on the protocol in the proposed ISO standard due to Blackburn and
Robshaw (which appeared at ACNS earlier this year).
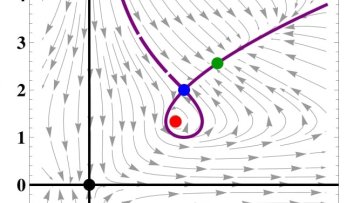
There is a wide class of problems in mathematics known as inverse problems. Rather than starting with a mathematical model and analysing its properties, mathematicians start with a set of properties and try to obtain mathematical models which display them. For example, in mathematical chemistry researchers try to construct chemical reaction systems that have certain predefined behaviours. From a mathematical point of view, this can be used to create simplified chemical systems that can be used as test problems for different mathematical fields.
Correctly predicting extinction is critical to ecology. Claim extinction too late, and you may be taking resources away from a species that actually could be saved. Claim extinction too early, and you may cause the true extinction due to stopping resources, such as removing protection of its habitat.
Alison Etheridge FRS, Professor of Probability in the University of Oxford, has been named Fellow of the Institute of Mathematical Statistics (IMS). Professor Etheridge received the award for outstanding research on measure-valued stochastic processes and applications to population biology; and for international leadership and impressive service to the profession.