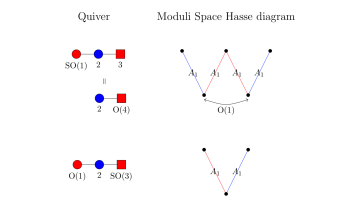
One of the main themes of geometry in recent years has been the appearance of unexpected dualities between different geometric spaces arising from ideas in mathematical physics. One famous such example is mirror symmetry. Another kind of duality, which I am currently investigating with collaborators from Oxford and Imperial College, is symplectic duality.
Many of the news stories on our website are about our research, and rightly so as it is an integral part of the academic life. But alongside research, academics are employed to teach, and to teach to the highest standard, providing a pipeline of graduates with the skills needed across the many sectors where they will work.
Knot theory studies embeddings of the circle into the three dimensional space and the first knot invariant was the Alexander polynomial. The world of quantum invariants started with the milestone discovery of the Jones polynomial and was expanded by Reshetikhin and Turaev’s algebraic construction which starts from a quantum group and leads to link invariants.
Learnable intra-layer feedback response in Spiking Neural Networks
[[{"fid":"63050","view_mode":"default","fields":{"format":"default"},"link_text":"Research_proposal___Learnable_feedback_response.pdf","type":"media","field_deltas":{"1":{"format":"default"}},"attributes":{"class":"media-element file-default","data-delta":"1"}}]]
Oxford Mathematician Lukas Brantner explains how generalised Lie algebras lead to new insights in Galois theory, deformation theory, and the theory of configuration spaces. Lukas has just been awarded a Royal Society University Research Fellowship.