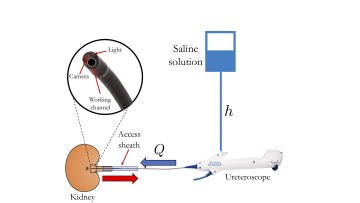
The discomfort experienced when a kidney stone passes through the ureter is often compared to the pain of childbirth. Severe pain can indicate that the stone is too large to naturally dislodge, and surgical intervention may be required. A ureteroscope is inserted into the ureter (passing first through the urethra and the bladder) in a procedure called ureteroscopy. Via a miniscule light and a camera on the scope tip, the patient’s ureter and kidney are viewed by a urologist.
the IceCube detector
flux with 8 years of IceCube data
14:15
Lie algebras in finite and mixed characteristic.
Abstract
Partition Lie algebras are generalisations of rational differential graded Lie algebras which, by a recent result of Mathew and myself, govern the formal deformation theory of algebro-geometric objects in finite and mixed characteristic. In this talk, we will take a closer look at these new gadgets and discuss some of their applications in algebra and topology
Wrinkling of Elastic Bilayers
Abstract
Wrinkling is a universal instability occurring in a wide variety of engineering and biological materials. It has been studied extensively for many different systems but a full description is still lacking. Here, we provide a systematic analysis of the wrinkling of a thin hyperelastic film over a substrate in plane strain using stream functions. For comparison, we assume that wrinkling is generated either by the isotropic growth of the film or by the lateral compression of the entire system. We perform an exhaustive linear analysis of the wrinkling problem for all stiffness ratios and under a variety of additional boundary and material effects.
16:00
The Nielsen-Thurston theory of surface automorphisms
Abstract
I will give an overview of the Nielsen-Thurston theory of the mapping class group and its connection to hyperbolic geometry and dynamics. Time permitting, I will discuss the surface entropy conjecture and a theorem of Hamenstadt on entropies of `generic' elements of the mapping class group. No prior knowledge of the concepts involved is required.
15:30
How many real Artin-Tate motives are there?
The goals of my talk are 1) to place this question within the framework of tensor-triangular geometry, and 2) to report on joint work with Paul Balmer (UCLA) which provides an answer in this framework.
A classical introduction to derived deformation theory of representations I
16:00
Holonomic D-modules, b-functions, and coadmissibility
Abstract
Since differentiation generally lowers exponents, it is straightforward that the space of Laurent polynomials $\mathbb{C}[x, x^{-1}]$ is a finitely generated module over the ring of differential operators $\mathbb{C}[x, \mathrm{d}/\mathrm{d}x]$. This innocent looking fact has been vastly generalized to a statement about holonomic D-modules, using the beautiful theory of b-functions (or Bernstein—Sato polynomials). I will give an overview of the classical theory before discussing some recent developments concerning a $p$-adic analytic analogue, which is joint work with Thomas Bitoun.
16:00
An overview of the SYZ conjecture
Abstract
The Strominger-Yau-Zaslow (SYZ) conjecture postulates that mirror dual Calabi-Yau manifolds carry dual special Lagrangian fibrations. Within the study of Mirror Symmetry the SYZ conjecture has provided a particularly fruitful point of convergence of ideas from Riemannian, Symplectic, Tropical, and Algebraic geometry over the last twenty years. I will attempt to provide a brief overview of this aspect of Mirror Symmetry.