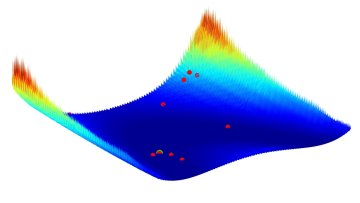
Harmonic map equations are an elliptic PDE system arising from the  
minimisation problem of Dirichlet energies between two manifolds. In  
this talk we present some some recent works concerning the symmetry  
and stability of harmonic maps. We construct a new family of  
''twisting'' examples of harmonic maps and discuss the existence,  
uniqueness and regularity issues. In particular, we characterise of  
singularities of minimising general axially symmetric harmonic maps,  
and construct non-minimising general axially symmetric harmonic maps  
with arbitrary 0- or 1-dimensional singular sets on the symmetry axis.  
Moreover, we prove the stability of harmonic maps from $\mathbb{B}^3$  
to $\mathbb{S}^2$ under $W^{1,p}$-perturbations of boundary data, for  
$p \geq 2$. The stability fails for $p <2$ due to Almgren--Lieb and  
Mazowiecka--Strzelecki.

(Joint work with Prof. Robert M. Hardt.)

This week is a women's only week in which we are joined by the Mirzakhani society, the society for undergraduate women in maths, for lunch.

We are very excited to have another session with invited speakers joining us for the lunch next week. Annika Heckel, Frances Kirwan and Marc Lackenby will all be joining us for a panel discussion on balancing family with academia. All are welcome to join us and to ask questions. 

We hope to see many of you at the lunch - Monday 1-2pm Quillen Room (N3.12).

The Oxford-Princeton Workshops on Financial Mathematics & Stochastic Analysis have been held approximately every eighteen months since 2002, alternately in Princeton and Oxford. They bring together leading groups of researchers in, primarily, mathematical and computational finance from Oxford University and Princeton University to collaborate and interact. The series is organized by the Oxford Mathematical and Computational Finance Group, and at Princeton by the Department of Operations Research and Financial Engineering and the Bendheim Center for Finance.

The classical Dirac operator is part of an osp(1|2) realisation inside the Weyl-Clifford algebra which is Pin-invariant. This leads to a multiplicity-free decomposition of the space of spinor-valued polynomials in irreducible modules for this Howe dual pair. In this talk we review an abstract generalisation A of the Weyl algebra that retains a realisation of osp(1|2) and we determine its centraliser algebra explicitly. For the special case where A is a rational Cherednik algebra, the centralizer algebra provides a refinement of the previous decomposition whose analogue was no longer irreducible in general. As an example, for the  group S3 in specific, we will examine the finite-dimensional irreducible modules of the centraliser algebra.