Graphs Solutions
Part of the Oxford MAT Livestream.
Revision Questions
1. Note that $x^2+4x+3=(x+3)(x+1)$. The graph of $y=f(x+2)$ is the graph of $y=f(x)$ after it has been translated two units to the left.
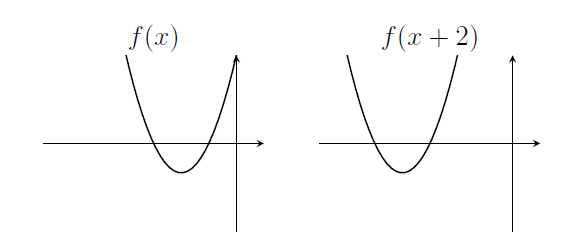
For $y=3f(2x)$, the graph is "squashed" by a factor of 2 parallel to the $x$-axis, then "stretched" by a factor of 3 parallel to the $y$-axis.
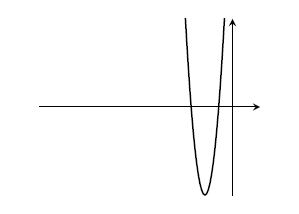
For $y=2 f( 3 x)$, the graph is squashed by a factor of 3 parallel to the $x$-axis, then stretched by a factor of 2 parallel to the $y$-axis

It's not the same as the previous graph. For example, the roots aren't in the same places.
$g(x)=x$ works for the last part; then $y=20x$ in both cases.
2. The graph of $y=f(x)$ and the graph of $y=2f(x+1)$;
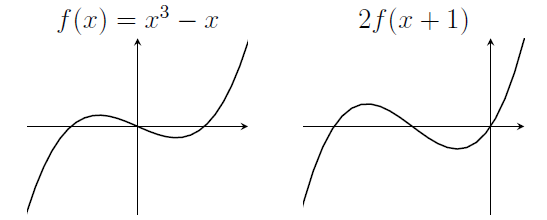
The graph of $y=2f(x)+1$;
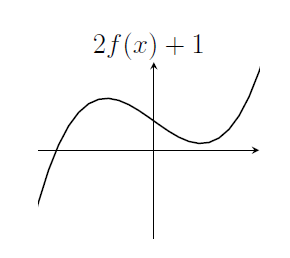
It's not the same as the previous graph. We could compare, for example, the values of $2f(x+1)$ and $2f(x)+1$ when $x=0$.
$g(x)=x/3+c$ for any constant $c$ works for the last part.
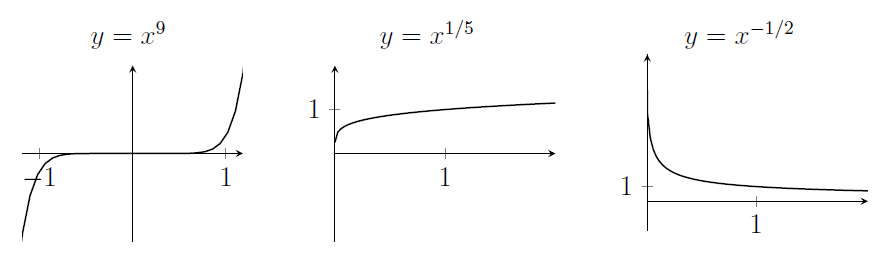
3. For large $n$, $y=x^n$ is close to zero between $-1$ and $1$. For small positive $n<1$, $y=x^n$ is approximately $1$ between $0$ and $1$. For negative $n$, the graph increases to infinity near $x=0$.
4. Note that $\sqrt{4x+1}=2\sqrt{x+\frac{1}{4}}$ so this is a translation of the graph of $y=\sqrt{x}$ by $\frac{1}{4}$ units in the negative $x$-direction followed by a stretch parallel to the $y$-axis with scale factor 2.
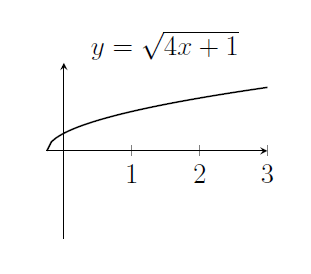
5. Note that $\log_2(x^2-2x+1)=\log_2((x-1)^2)$.

6. The function $\sin(x^2)=0$ when $x^2= 180^\circ n$ for $n$ a whole number, so the graph crosses the $x$-axis more and more frequently as $x$ increases. The graph has reflectional symmetry in the $y$-axis.
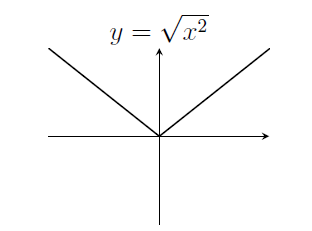
7. If $x\geq0$ then $\sqrt{x^2}=x$ but if $x<0$ then $\sqrt{x^2}=-x$, because $\sqrt{u}$ is always the positive root.
8. The graph of $y=2^x$, and the graph of $y=2^{-x}$.
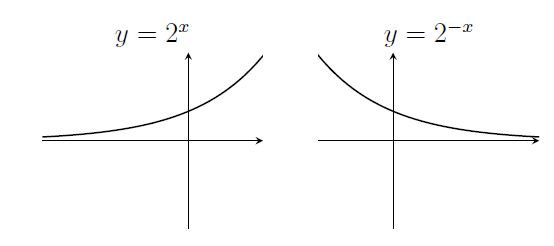
One is the reflection of the other, reflecting in the $y$-axis.
9. The graph of $y=\cos 2x$, and the graph of $y=\frac{1}{2} + \frac{1}{2}\cos 2x$.

10. The equation $y=4-x$ is the equation of a straight line.
The equation $y=4-x^2$ is the equation of a parabola.
The equation $x^2=4-y^2$ is the equation of a circle with radius 2.
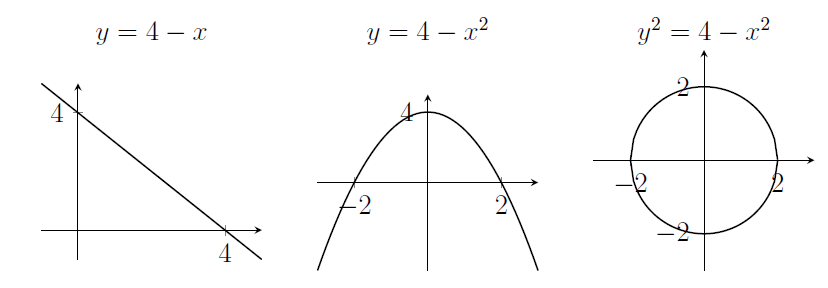
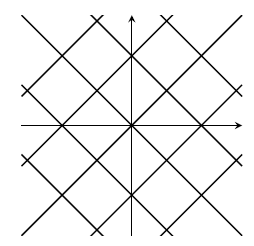
11.. If $\cos x = \cos y$ then either $x=y$, or $x=y+360^\circ n$ for some whole number $n$, or $x+y=360^\circ n$ for some whole number $n$. These are the equations of lines.
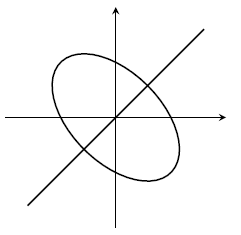
12. It's possible that $x=y$, but because $f(x)=x^3-x$ is not a one-to-one function, there are other points $(x,y)$ that we must consider. For some values of $c$ there are three solutions to $x^3-x=c$.
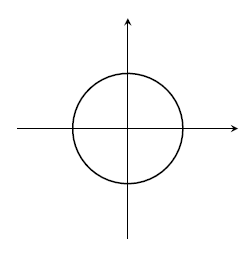
13. The equation $x^4+2x^2y^2+y^4-3x^2-3y^2+2=0$ simplifies to $(x^2+y^2)^2-3(x^2+y^2)+2=0$, which is a quadratic for $x^2+y^2$, with roots $x^2+y^2=1$ or $x^2+y^2=2$. This is a pair of circles.

14. The equation $x^6+3x^4y^2+3x^2y^4+y^6=1$ simplifies to $(x^2+y^2)^3=1$ so we have $x^2+y^2=1$ and this is a circle.
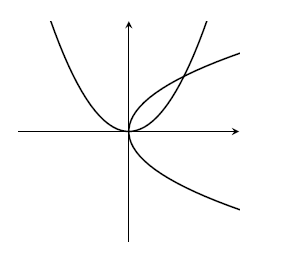
15. The equation rearranges to $x^3-xy=x^2y^2-y^3$. Take out a factor of $x$ on the left and $y^2$ on the right to factorise this as $x(x^2-y)=y^2(x^2-y)$ so either $x^2=y$ or $x=y^2$. This is a pair of parabolas.
MAT 2008 Q3
(i) The graph $y=f(-x)$ represents a reflection in the $y$-axis.
The graph $y=f(x-1)$ represents a translation by 1 unit in the positive $x$-direction.
The graph $y=-f(x)$ represents a reflection in the $x$-axis.
Trying these on the graph that we're given for $y=f(x)$, I get something that looks like $(A)$ or $(B)$ for the first and third transformations, while the graph $(C)$ is clearly the translation. Looking at the axes, I can see that $(A)$ is the reflection in the $x$-axis.
So $y=f(-x)$ is $(B)$, $y=f(x-1)$ is $(C)$, and $y=-f(x)$ is $(A)$.
(ii) Note that $-x^2\leq 0$, and gets very negative very quickly as $x$ gets large (no matter whether $x>0$ or $x<0$). We also know that, if $u$ is very negative, then $2^u$ is close to zero. The graph will have reflectional symmetry in the $y$-axis, and the $y$-intercept is $1$.
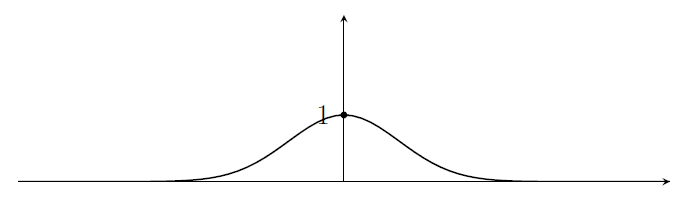
The maximum value of $2^{-x^2}$ comes where $-x^2$ is maximised, which happens when $x=0$. The stationary point here has coordinates $(0,1)$.
Now note that $2x-x^2=-(x-1)^2+1$ if we complete the square. So
$$
2^{2x-x^2}=2\times 2^{-(x-1)^2}
$$
This is a translation by 1 unit in the positive $x$-direction, followed by a stretch parallel to the $y$-axis with scale factor 2. The $y$-intercept is 1 again, so the graphs intersect there. After the translations, the stationary point is now at $(1,2)$. My sketch now looks like this;
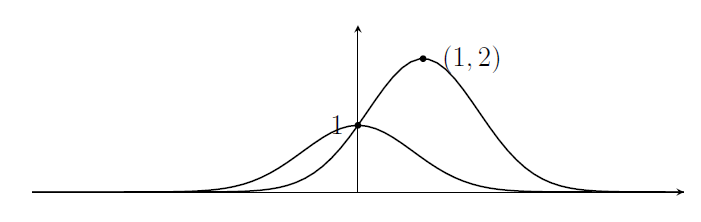
(iii) Note that $2^{-(x-c)^2}$ is just a translation of $2^{-x^2}$. We want the area under the graph between 0 and 1 to be large, so we want the big bit of the graph to be in that range. Let's park it right in the middle with $c=\frac{1}{2}$.
Extension
- Things that are unchanged; number of turning points, egree of the polynomial (if they're polynomials), and more.
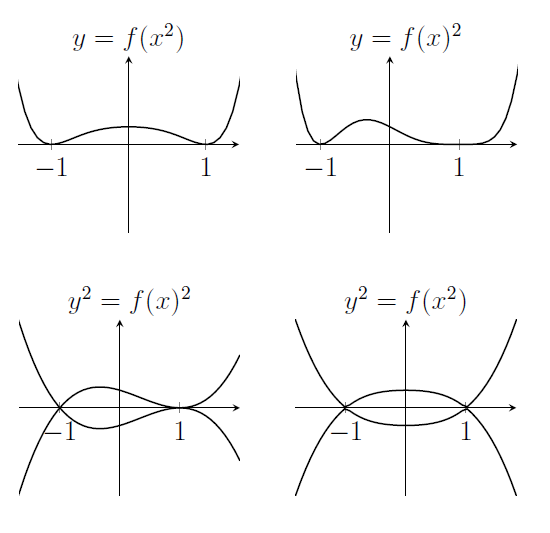
Things that might change; number of roots, behaviour for large $x$, $y$-intercept, value at $x=37$, and more. - The graph of $f(x^2)$ just uses the part of the graph of $y=f(x)$ where $x\geq 0$, and there's some distortion. For this function $f(x)$, the values happen to be positive in $x\geq 0$. This graph will have reflectional symmetry in the $y$-axis.
The graph of $y=f(x)^2$ takes values of $f(x)$ and squares them, so it'll definitely get a positive result. There's no obvious symmetry to this graph.
If $y^2=f(x)^2$ then either $y=f(x)$ or $y=-f(x)$. This graph will have reflectional symmetry in the $x$-axis.
For $y^2=f(x^2)$ we have to take our previous graph of $f(x^2)$ and imagine taking the square root of the values, and also the negative of that. This graph will have reflectional symmetry in both the $x$-axis and the $y$-axis.
MAT 2011 Q4
(i) These are straight lines.
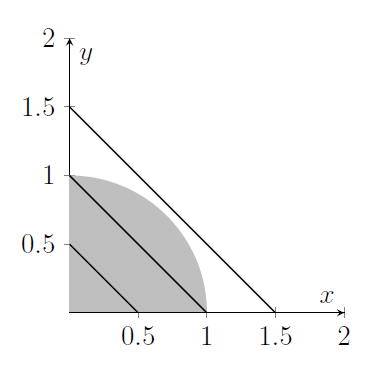
The maximum value of $x+y$ would come if we made the line tangent to the circle $x^2+y^2=1$. By reflectional symmetry in the line $y=x$, this happens at the point where $x=y$, which is where $2x^2=1$ so $x=y=2^{-1/2}$. The value of $x+y$ there is $\sqrt{2}$.
(ii) These are hyperbolas.

We can make $x^2+y^2+4xy$ large if we can make $x^2+y^2$ and $4xy$ large at the same time. This is possible, if we take the point $x=y=2^{-1/2}$ again (there's a reflectional symmetry again). The value there is
$$
x^2+y^2+4xy=1+4\times 2^{-1/2}\times 2^{-1/2}=3
$$
We can make $x^2+y^2-6xy$ large if we can make $x^2+y^2$ and $6xy$ small at the same time. This is possible, if we take a point on either axis that's also on the circle. Then the value of $xy$ is zero, so $x^2+y^2-6xy=1$.
(iii) We have $(x-2)^2+(y-1)^2=k+5$ which is a circle centered on $(2,1)$ with radius $\sqrt{k+5}$.
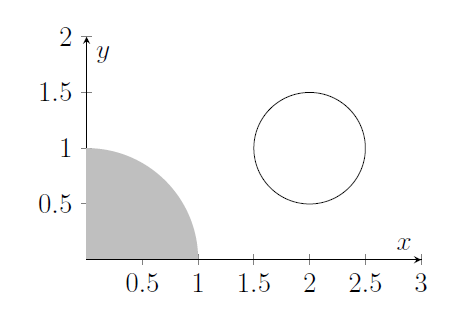
We want a small value for $k$, so a small circle, but we need some point in $Q$ to be on this circle. If we make this circle just large enough to touch the boundary of $Q$, then that's the smallest $k$ we can get away with.
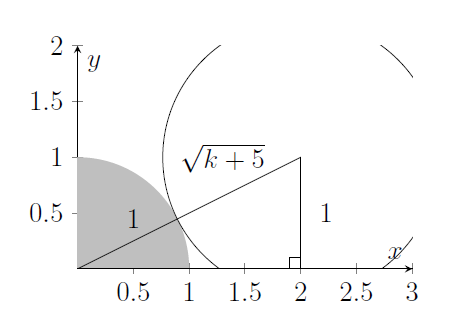
There's a right-angled triangle in that picture, and Pythagoras gives
$$
2^2+1^2=(1+\sqrt{k+5})^2
$$
which rearranges to $k=1-2\sqrt{5}$ (taking the smaller root).
Extension
- Join the centres. Check if the sum of the radii is less than the distance between the centres. If it is, then find the intersection points between the line and each circle. Check the distances between those points. If the sum of the radii is too big, it might be the case that the circles intersect, or that one lies inside the other. Each of these cases can be detected by looking at the radii and the distance between the centres of the circles.
- The picture is still symmetric in the line $y=x$, and the maximum occurs at $(2^{-1/4},2^{-1/4})$. The value of $x^2+y^2+4xy$ there is $3\sqrt{2}$.

