Solutions

Part of the Oxford MAT Livestream

These are the solutions for the Geometry worksheet. You don't need these unless you're really really stuck, or if you've already done the problems and want to see how I did them.

Warm-up

1. Draw a diagram to show the three separate position vectors $\displaystyle \binom{3}{2}$ and $\displaystyle\binom{-4}{1}$ and $\displaystyle\binom{1}{-2}$.

  • Something likeThree arrows on a grid. One points three squares right and two squares up. One points one square left and two squares down. One points four squares left and one square up

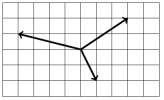
2. Add the vectors $\displaystyle \binom{3}{2}$ and $\displaystyle \binom{-4}{1}$. Show this on your diagram.

  • We add the components separately, so $\displaystyle \binom{3}{2}+\binom{-4}{1}=\binom{-1}{3}$.
  • My diagram now looks like this.Same diagram, but with an arrow pointing one square left and three squares up. A dotted construction line goes from the end of the (3,2) vector to the end of this new vector.

     

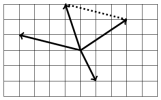
3. Find $\displaystyle 3 \binom{-4}{1}+2\binom{1}{-2}$. Show this on your diagram.

  • You multiply a vector by a scalar by multiplying each component, so $\displaystyle 3 \binom{-4}{1}=\binom{-12}{3}$ and $\displaystyle 2\binom{1}{-2}=\binom{2}{-4}$. Then add them together $\displaystyle \binom{-12}{3}+\binom{2}{-4}=\binom{-10}{-1}$.
  • My diagram now looks like this.The same diagram again, but with a new arrow pointing ten squares left and one square down. A dotted construction line goes in the direction of the (-4,1) vector for three times the distance, then turns sharply and continues parallel to the (1,-2) vector for twice the length of that vector, ending at the point of this newest arrow.

     

4. Find the equation of the line through the points $(1,5)$ and $(3,-1)$.

  • This line has gradient $(-1-5)/(3-1)=-3$ and goes through $(1,5)$ so it's $y-5=-3(x-1)$ which can also be written as $y=8-3x$.

5. Find the equation of the line through the point $(3,5)$ with gradient 2.

  • This must be $y=2x+c$ for some constant $c$, and the line goes through $(3,5)$ so $5=6+c$ and so the line is $y=2x-1$.

6. Find equations of three lines such that the region bounded by the three lines is an equilateral triangle.

  • There are lots of examples that work! I decided to use the $x$-axis as one of my lines (that's $y=0$), and then use something like $y=\sqrt{3}-ax$ and $y=\sqrt{3}+bx$ for some $a$ and $b$; I've chosen those $y$-intercepts so that $(0,\sqrt{3})$ is a corner of the triangle (I've drawn a little sketch of an equilateral triangle with side-length 2 and height $\sqrt{3}$ which I'm aiming for).
  • I need those two lines to go through $(\pm 1,0)$. I can do that by choosing $a$ and $b$ carefully, and I end up with the three lines $y=0$ and $y=\sqrt{3}(1-x)$ and $y=\sqrt{3}(1+x)$.

7. A circle has centre $(-1,4)$ and radius 3. Write down an equation for the circle. What's the area of this circle?

  • $(x+1)^2+(y-4)^2=3^2$
  • The area is $\pi r^2$ and $r=3$ so the area is $9\pi$.

8. A circle is given by $x^2+9x+y^2-3y=10$. Find the centre and radius of the circle.

  • $x^2+9x+y^2-3y=\left(x+\frac{9}{2}\right)^2+\left(y-\frac{3}{2}\right)^2-\frac{81}{4}-\frac{9}{4}$. The equation of the circle is $\left(x+\frac{9}{2}\right)^2+\left(y-\frac{3}{2}\right)^2=10+\frac{90}{4}$.
  • So the centre is $\left(-\frac{9}{2},\frac{3}{2}\right)$ and the radius is $\sqrt{\frac{65}{2}}$.

9. Points $A$ and $B$ lie on a circle with centre $O$ and radius 2. The angle $\angle AOB$ is $120^\circ$. Find the length of the arc between $A$ and $B$. Find the area enclosed by that arc and the radii $OA$ and $OB$.

  • A circle with two radii marked, crossing the circle at A and B.
  • Since $120^\circ$ is one-third of $360^\circ$, the length of the arc is one-third of the length of the circumference $2\pi r$ with $r=2$. So the length of the arc is $\frac{4}{3}\pi$.
  • The area is one-third of $\pi r^2$, which works out to be $\frac{4}{3}\pi$.

10. A circle is given by $x^2+y^2=4$. The line $x=1$ splits the circle into two regions. Find the area of each region.

  • A circle with a vertical line crossing it on the right. Radii are drawn from the centre of the circle to the points of intersection.
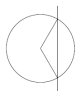
  • First let's find the points of intersection. We have $x=1$ so $y^2=3$ so $y=\pm \sqrt{3}$. Now find the angle at the centre between the radii. There's a triangle here with width $1$ and height $2\sqrt{3}$. Some trigonometry gives the angle at the centre as $120^\circ$.
  • We want the area of each region. The area of the small region ($x\geq1$) is a sector of the circle minus the area of the triangle described above. That's $\frac{4}{3}\pi - \sqrt{3}$
  • The larger region is made of a larger sector plus the triangle, but let's just use the fact that the total area is $4\pi$ and subtract the area of the small region to get $\frac{8}{3}\pi+\sqrt{3}$.

11. Two circles are given by $x^2+y^2=4$ and $(x-2)^2+y^2=4$. Find the area of the region that's inside both circles.

  • Two overlapping circles with a vertical line through the two points of intersection.
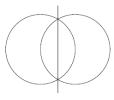
  • This looks really hard, but luckily we've already done most of the work.
  • Find the points of intersection. $x^2=(x-2)^2$ so $x=2-x$ or $x=x-2$, which only has $x=1$ as a solution. The $y$-coordinates are the same as in the previous question; the line $x=1$ perfectly splits the area we're looking for down the middle and the situation is symmetric.
  • So we just need to double the small area we found before.
  • That's $\frac{8}{3}\pi -2\sqrt{3}$.

12. The points $(0,0)$ and $(1,a)$ and $(0,a+1/a)$ all lie on the same circle. Find the centre of the circle in terms of $a$.

  • Two line segments that share an end-point are shown, with the angle between them marked as a right angle. The ends of the line segments all lie on a circle.
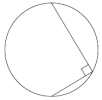
  • We could write down equations for the distance of a general point $(x,y)$ to each of these points and set them equal to each other, but that's a lot of work.
  • Instead, note that the gradient of the line from $(0,0)$ to $(1,a)$ is $a$ and the gradient of the line from $(1,a)$ to $(0,a+1/a)$ is $-1/a$. These gradients multiply to $-1$, so the lines are at right-angles.
  • The angle in a semi-circle is a right-angle, so the line from the first point to the third point is the diameter of the circle.
  • The centre is at the midpoint of the diameter, so it's at $(0,a/2+1/2a)$.

MAT questions

MAT 2013 Q1H

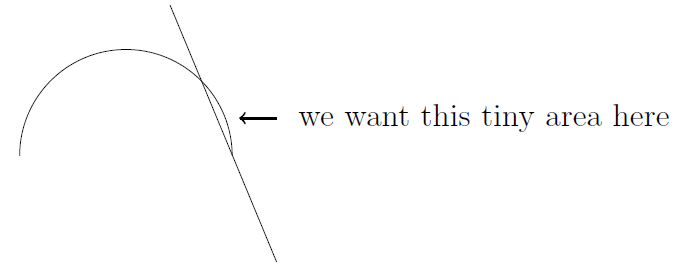
  • First, note that the first graph is a semi-circle (the upper half of $x^2+y^2=2$) and the second graph is a straight line.
  • Inspired by this observation, let's find the intersection points and the angle at the centre (like the warm-up problem).
  • The points of intersection are $(\sqrt{2},0)$ and $(1,1)$, and the angle at the centre between radii to these points is $45^\circ$. So the area between the graphs is one-eighth of a circle minus the area of a triangle made by the radii and the line.
  • This area is therefore \[\frac{1}{8}\pi \times (\sqrt{2})^2 - \frac{1}{2}\sqrt{2}\times \sqrt{2}\times \frac{\sqrt{2}}{2}=\frac{\pi}{4}-\frac{\sqrt{2}}{2}.\]
  • The answer is (b)

MAT 2017 Q1G

A point is marked inside a circle. A two lines through that point are shown with a big arrow arcing from one line to the other labelled theta
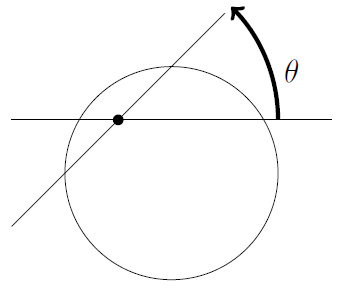
  • This line goes through the point $(-1,1)$ which is inside the circle $x^2+y^2=4$. The gradient of the line is $\tan\theta$ so the angle between the line and the $x$-axis is $\theta$.
  • As we change $\theta$, the areas change size. The maximum comes when the line is at right-angles to the radius that goes through $(-1,1)$.
  • This actually happens for two values of $\theta$ because $\theta+180^\circ$ gives the same line as $\theta$.
  • The answer is (b).

MAT 2016 Q1I

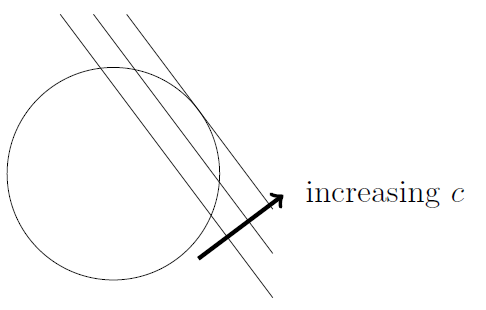
  • Note that $ax+by$ takes the value $c$ on the line $ax+by=c$. We want to make $c$ big while still having the line intersect the circle for at least one point. We can do this in an extreme way by making the line tangent to the circle.
  • Time for some geometry; the gradient of the line is $-a/b$ and the radius is at right angles to the tangent so the radius has gradient $b/a$. Intersecting the radius $y=bx/a$ with the circle $x^2+y^2=1$ gives the coordinates of the point of tangency as $(a,b)/\sqrt{a^2+b^2}$, and at that point the value of the function we're maximising is $\sqrt{a^2+b^2}$.
  • The answer is (c).

MAT 2016 Q4

Three circles in a row are tangent to each other. They all sit on a horizontal line, and there's a diagonal line that's tangent to all three circles. The angle between the two lines is 2 times alpha

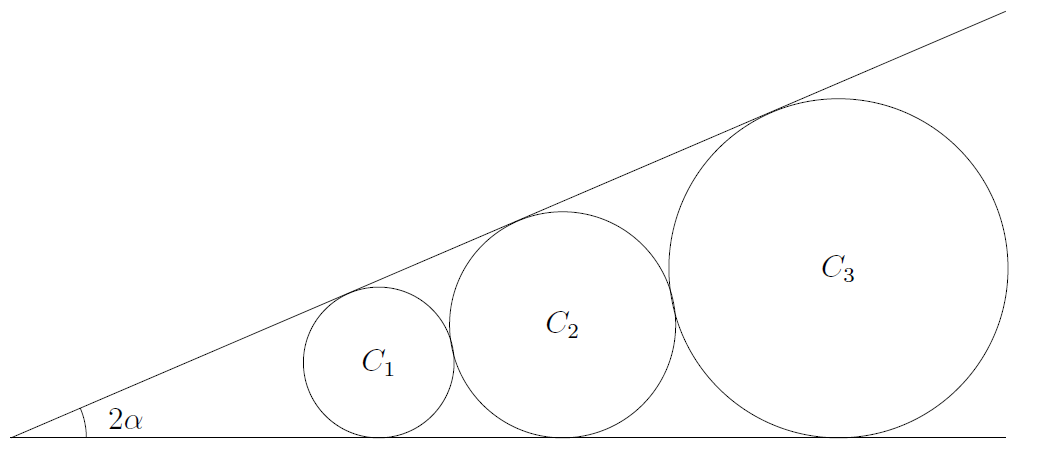
Not to scale at all

(i) First we're just asked about $C_1$.

The first circle is tangent to both lines. The radius to the x-axis is labelled 1, and the line from the centre of the circle to the origin is drawn, making an angle alpha with the x-axis at the origin.

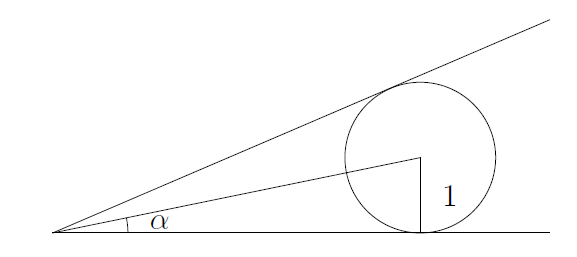
The $y$-coordinate of the center is clearly $1$. There's a right-angled triangle here, and some trigonometry gives the $x$-coordinate of the centre as $1/\tan(\alpha)$.

(ii) The radius of $C_1$ is 1, so the equation for the circle is \[\left(x-\frac{1}{\tan\alpha}\right)^2+\left(y-1\right)^2=1.\]

(iii) Now we're asked about the pair $C_1$ and $C_2$.

Two circles are tangent to two lines. The line between the centres is shown (it goes through the point of tangency). A horizontal line from the centre of the smaller circle is shown. These form a triangle with part of the radius of the larger circle.

I've drawn in a line parallel to the $x$-axis through the centre of $C_1$ so that I can label the angle $\alpha$, the thing I'm trying to solve for. This gives me a little right-angled triangle where I know two of the side lengths, and I would like to know one of the angles. I know the hypotenuse of the triangle because the circles just touch (so the radii are both perpendicular to the shared tangent and form a straight line), giving a distance of 4 between the centres. The side of the triangle opposite $\alpha$ is clearly just the difference between the radii, which is 2. So $\sin \alpha=\frac{1}{2}$ so $\alpha=30^\circ$ (noting that $0<\alpha<45^\circ$).

(iv) We can draw a very similar diagram to the previous part, and write $R$ for the radius of $C_3$. Then we have $\sin 30^\circ=(R-3)/(R+3)$. This rearranges to give $R=9$. The radii of circles $C_1$ and $C_2$ and $C_3$ form a geometric sequence!

(v) We are asked about the area between circles $C_1$ and $C_2$ and the $x$-axis.

The area between the two circles is shaded. The x-axis, the radii of each circle, and the line joining their centres form a trapezium. The angles are 120 and 60 at the centres of the circles.

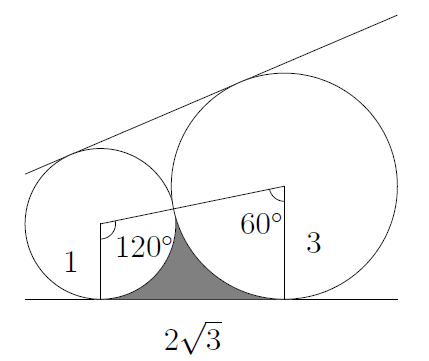
This is the area of a trapezium (a rectangle plus a triangle) minus the area of two sectors of circles. We know the $x$-coordinate of the centre of $C_1$ is $1/\tan\alpha=\sqrt{3}$. Similarly, the centre of $C_2$ has $x$-coordinate $3\sqrt{3}$ (which is why I've labelled the base of the trapezium as $2\sqrt{3}$). The area we want is \[2\times 2\sqrt{3}-\frac{1}{3}\pi-\frac{1}{6}\pi \times 3^2=4\sqrt{3}-\frac{11\pi}{6}\]

 

Reflection

  • Drawing pictures is generally helpful. I think that there's a bit of my brain that's good at recognising shapes, and I can use that to spot how to break down complicated areas into parts maybe (I am not a neuroscientist).
  • Drawing the right diagram makes geometry questions like this easier. Sometimes it can feel like "I don't know which line to draw", but in the long MAT question above, the lines that we drew were motivated by either being radii of the circles (known lengths), or joining the centres of touching circles (interesting because the circles just touch), or parallel to the $x$-axis (so that I could mark in $\alpha$).
  • I like to imagine things moving as parameters change.
Please contact us with feedback and comments about this page. Last updated on 25 Aug 2022 11:51.