The Aldous diffusion
Abstract
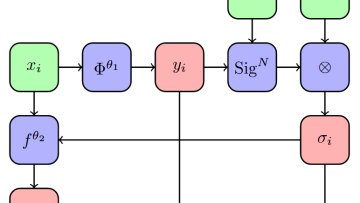
The Aldous diffusion is a conjectured Markov process on the
space of real trees that is the continuum analogue of discrete Markov
chains on binary trees. We construct this conjectured process via a
consistent system of stationary evolutions of binary trees with k
labelled leaves and edges decorated with diffusions on a space of
interval partitions constructed in previous work by the same authors.
This pathwise construction allows us to study and compute path
properties of the Aldous diffusion including evolutions of projected
masses and distances between branch points. A key part of proving the
consistency of the projective system is Rogers and Pitman’s notion of
intertwining. This is joint work with Noah Forman, Soumik Pal and
Douglas Rizzolo.