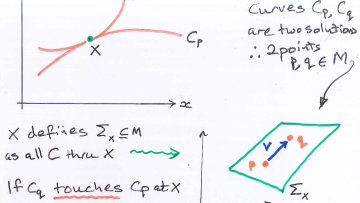
The core idea behind metric spaces is the triangular inequality. Metrics have been generalized in many ways, but the most tempting way to alter them would be to "flip" the triangular inequality, obtaining an "anti-metric". This, however, only allows for trivial spaces where the distance between any two points is 0. However, if we intertwine the concept of antimetrics with the structures of partial linear--and cyclic--orders, we can define a structure where the anti-triangular inequality holds conditionally. We define this structure, give examples, and show an interesting result involving metrics and antimetrics.

There have been two main attempts so far to forecast the level of development of artificial intelligence (or ‘computerisation’) over time, Frey and Osborne (2013, 2017) and Manyika et al (2017). Unfortunately, their methodology seems to be flawed. Their results depend upon expert predictions of which occupations will be automatable in 2050, but these predictions are notoriously unreliable. Therefore, we develop an alternative which does not depend upon these expert predictions. We build a dataset of all the start-ups, firms, and university research laboratories working on automating different types of tasks, and use this to build a dynamic network model of them and how they interact. How automatable each type of task is ‘emerges’ from the model. We validate it, predicting the level of development of supervised learning in 2017 using data from the year 2000, and use it to forecast of the automatability of each of these task types from 2018 to 2050. Finally, we discuss extensions for our model; how it could be used to test the impact of public policy decisions or forecast developments in other high-technology industries.

As a rule of thumb, the dominant resistive force on a cyclist riding along a flat road at a speed above 10mph is aerodynamic drag; at higher speeds, this drag becomes even more influential because of its non-linear dependence on speed. Reducing drag, therefore, is of critical importance in bicycle racing, where winning margins are frequently less than a tyre's width (over a 200+km race!). I shall discuss a mathematical model of aerodynamic drag in cycling, present mathematical reasoning behind some of the decisions made by racing cyclists when attempting to minimise it, and touch upon some of the many methods of aerodynamic drag assessment.

In this talk we will introduce quantifier elimination and give various examples of theories with this property. We will see some very useful applications of quantifier elimination to algebra and geometry that will hopefully convince you how practical this property is to other areas of mathematics.