Research
Our Research
Our research covers the spectrum of fundamental and applied mathematics.
Below are the various research groups through which you can explore these areas in more detail. We also host the Clay Mathematics Institute.
Also see our case studies and films that spotlight different aspects of our research and our list of faculty books.
Other Resources
Algebra
Algebraic techniques are of central importance in modern mathematics. As such the algebra group sits naturally among a number of major research topics in the department, with connections to geometric topology via group theory, homotopy theory and number theory through representation theory, and algebraic geometry through geometric representation theory.
Combinatorics
Research interests: extremal combinatorics, graph theory, and combinatorial number theory.
Functional Analysis

Research interests: operator theory, including unbounded operators, and abstract differential equations.
Geometry
Research includes algebraic geometry, Riemannian geometry, homological mirror symmetry and symplectic geometry. There are links with representation theory in the algebra group.
History of mathematics

History of mathematics is a multidisciplinary subject with close ties to the History Faculty. Research interests cover mathematics and its social context from the early modern period right up to the twentieth century.
Logic
Research interests: analytic topology, geometric stability theory, and the model theory of p-adic fields and diophantine geometry.
Machine Learning and Data Science

Machine Learning and Data science are being developed using wide ranging mathematical techniques. Our particular research expertise include: applied and computational harmonic analysis, networks, optimisation, random matrix theory, rough paths, topological data analysis, and the application of these methods.
Mathematical & Computational Finance
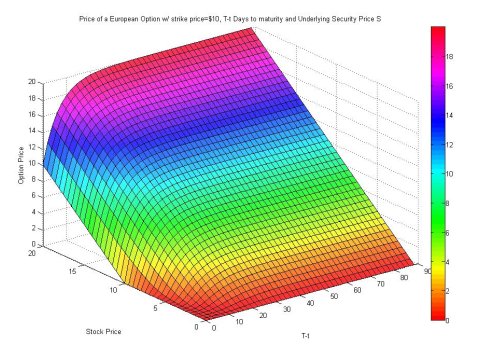
The Mathematical and Computational Finance Group is one of the world's leading research group in the area of mathematical modelling in finance. Research topics include derivative pricing, computational methods, credit risk, quantitative risk management, market microstructure and high-frequency modelling, macro-financial modelling and systemic risk.
Mathematical Biology
Development and application of mathematical and computational methodologies for the understanding of key problems in the biological and medical sciences.
Mathematical Physics
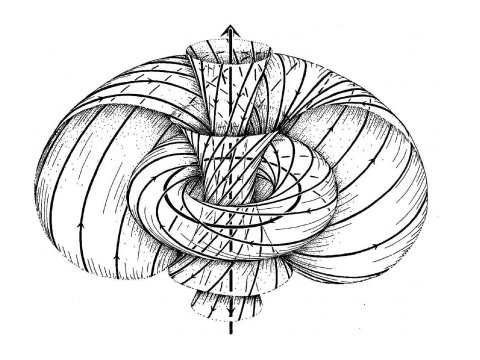
Research interests: gauge and gravity theories (quantum field theories), string theory, twistor theory, Calabi-Yau manifolds, quantum computation and cryptography.
Number theory
Members of the number theory group work in analytic and combinatorial number theory, arithmetical algebraic geometry, and computational number theory, with numerous and deep connections to current issues in algebra, combinatorics, geometry, topology, logic, and mathematical physics.
Numerical analysis

The numerical analysis group develops and analyses algorithms for mathematical problems related to partial differential equations, linear algebra, optimization and other areas. The is a strong involvement in applications, with particularly close connections with OCIAM, the Wolfson Centre for Mathematical Biology, and the Centre for Nonlinear PDE.
Oxford Centre for Industrial and Applied Mathematics
![]()
Research interests: energy, industry, geoscience, networks, finance, methodologies.
Oxford Centre for Nonlinear Partial Differential Equations

Research focuses on the fundamental analysis of nonlinear PDE, and numerical algorithms for their solution. Current areas of interest include the calculus of variations, nonlinear hyperbolic systems, inverse problems, homogenization, infinite-dimensional dynamical systems, geometric analysis and PDE arising in solid and fluid mechanics, materials science, liquid crystals, biology and relativity.
Who's who in the Oxford Centre for Nonlinear Partial Differential Equations
Stochastic analysis
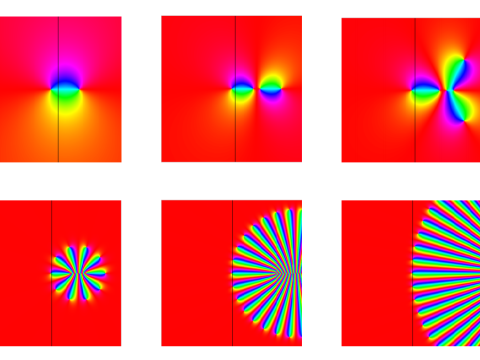
Research interests: rough path theory, Schramm-Loewner evolution, mathematical population genetics, financial mathematics, self-interacting random processes.
Topology
The members of the topology group have very wide ranging interests in algebraic, geometric and differential topology. Both high- and low-dimensional manifold theory (including knot theory) are represented. Particular research foci are topological quantum field theory and geometric group theory.


