Doctor of Philosophy (DPhil)
What is a DPhil?
A DPhil is Oxford's name for a PhD - a higher research degree which allows you to make an original contribution to mathematics in the form of a thesis. A DPhil takes at least three years to complete, and around two thirds of our postgraduate leavers go on into academia (according to the latest destination data). During your DPhil, you will be supervised by at least one academic, although some students will have more than one supervisor (particularly if they are working across disciplines). Unlike CDT courses (and PhDs in other countries), you will begin to do research straight away and there is no prescribed taught component. However, you are very welcome to attend seminars and you can choose from a wide variety of taught courses and skills training to enhance your broader mathematical knowledge and develop your career. There may also be journal clubs or seminar series specific to your area of study. If you enjoy doing mathematics, and would like to be part of a lively and world-class research institute, then you should take a look at our research groups to see if they align with your own interests.
All applications should be submitted online through the University's Graduate Application Form. To find out more about how to apply, see the how to apply page, or go to the University of Oxford's graduate application guide.
For information about scholarships and funding, see the University of Oxford's fees, funding, and scholarship search.
Key Deadlines
Funding deadlines for students applying for EPSRC and Departmental awards
- 5th January 2024 (12:00 GMT)
- 1st March 2024 (12:00 GMT)
Please apply by the 5th January deadline if you would like to be considered for any centrally administered funds. Further information regarding these funds can be found here.
Fees and Funding
Information on University fees and funding can be found here.
Scholarships
Wang Scholarship
The Wang Scholarship is available to DPhil candidates and provides full fees and a stipend for four years.
Charles Coulson Scholarship in Mathematical Physics
This award is available to DPhil candidates in Mathematical Physics and provides full fees and a stipend for four years.
Algebra
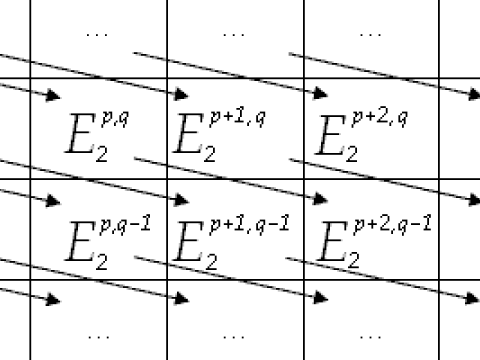
Research interests: group theory, representation theory and algebraic aspects of geometry.
Combinatorics
Research interests: extremal combinatorics, graph theory, and combinatorial number theory.
Geometry
Research interests: algebraic geometry, geometric representation theory, and differential geometry.
Research interests: operator theory, including unbounded operators, and abstract differential equations.
History of Mathematics
Research interests: history of algebra (19th and 20th century), history of modern algebra, and Soviet mathematics.
Logic
Research interests: analytic topology, geometric stability theory, and the model theory of p-adic fields and diophantine geometry.
Mathematical Biology
Research interests: cancer modelling, collective behaviour, gene regulatory networks, multiscale modelling, pattern formation, and sperm dynamics.
Mathematical & Computational Finance
Research interests: behavioural finance, financial big data, high dimensional numerical methods, stochastic analysis.
Mathematical Physics
Research interests: gauge and gravity theories (quantum field theories), string theory, twistor theory, Calabi-Yau manifolds, quantum computation and cryptography.
Number Theory
Research interests: analytic number theory, arithmetic geometry, prime number distribution, and Diophantine geometry.
Numerical Analysis
Research interests: complexity in optimisation, symmetric cone programming, numerical solutions of PDEs.
Oxford Centre for Industrial and Applied Mathematics
Research interests: energy, industry, geoscience, networks, finance, methodologies.
Topology
Research interests: geometric group theory, 3-manifold topology and knot theory, K-theory, algebraic topology.
Oxford Centre for Nonlinear Partial Differential Equations
Research interests: geometric analysis, inverse problems, nonlinear hyperbolic systems, specific PDE systems.
Stochastic Analysis
Research interests: rough path theory, Schramm-Loewner evolution, mathematical population genetics, financial mathematics, self-interacting random processes.
