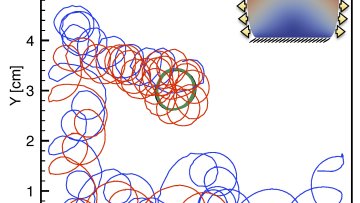
Cycling science is a lucrative and competitive industry in which small advantages are often the difference between winning and losing. For example, the 2017 Tour de France was won by a margin of less than one minute for a total race time of more than 86 hours. Such incremental improvements in performance come from a wide range of specialists, including sports scientists, engineers, and dieticians. How can mathematics assist us?
Oxford Mathematician Ilya Chevyrev talks about his research into using stochastic analysis to understand complex systems.
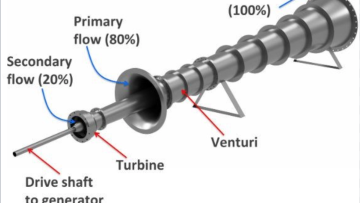
The generation of electricity from elevated water sources has been the subject of much scientific research over the last century. Typically, in order to produce cost-effective energy, hydropower stations require large flow rates of water across large pressure drops. Although there are many low head sites around the UK, including numerous river weirs and potential tidal sites, the pursuit of low head hydropower is often avoided because it is uneconomic.
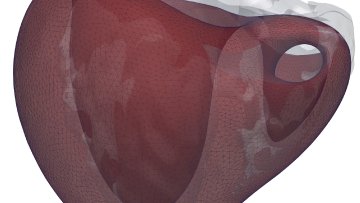
Oxford Mathematician Ricardo Ruiz Baier, in collaboration mainly with the biomedical engineer Alessio Gizzi from Campus Bio-Medico, Rome, have come up with a new class of models that couple diffusion and mechanical stress and which are specifically tailored to the study of cardiac electromechanics.
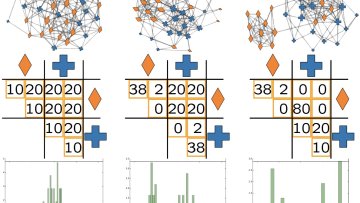
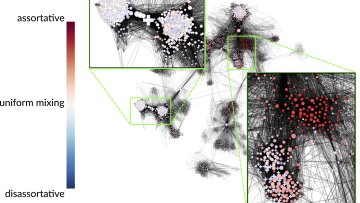
In this collaboration with researchers from the University of Louvain, Renaud Lambiotte from Oxford Mathematics explores the mixing of node attributes in large-scale networks.
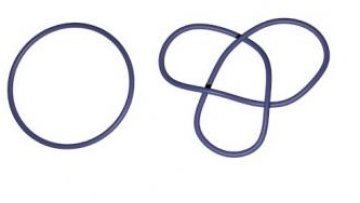
Knots are widespread, universal physical structures, from shoelaces to Celtic decoration to the many variants familiar to sailors. They are often simple to construct and aesthetically appealing, yet remain topologically and mechanically quite complex.
Knots are also common in biopolymers such as DNA and proteins, with significant and often detrimental effects, and biological mechanisms also exist for 'unknotting'.
Oxford Mathematician Andras Juhasz discusses and illustrates his latest research into knot theory.
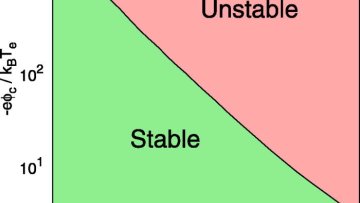
Oxford Mathematician John Allen, Professor Emeritus of Engineering Science, talks about his work on the electrohydrodynamic stability of a plasma-liquid interface. His collaborators are Joshua Holgate and Michael Coppins at Imperial College.
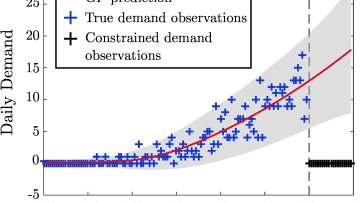
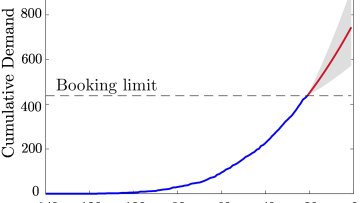
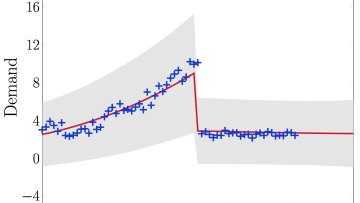
Oxford Mathematicians Ilan Price and Jaroslav Fowkes discuss their work on unconstraining demand with Gaussian Processes.
"One of the key revenue management challenges which airlines, hotels, cruise ships (and other industries) all share is the need to make business decisions in the face of constrained (or censored) demand data.
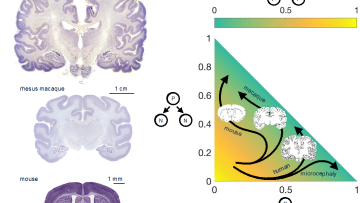
The brain is the most complicated organ of any animal, formed and sculpted over 500 million years of evolution. And the cerebral cortex is a critical component. This folded grey matter forms the outside of the brain, and is the seat of higher cognitive functions such as language, episodic memory and voluntary movement.
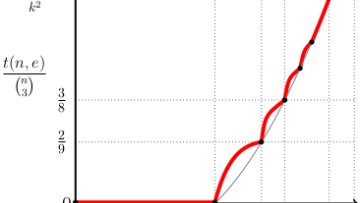
Oxford Mathematician Katherine Staden provides a fascinating snapshot of the field of combinatorics, and in particular extremal combinatorics, and the progress that she and her collaborators are making in answering one of its central questions posed by Paul Erdős over sixty years ago.
What does boiling water have in common with magnets and the horizon of black holes? They are all described by conformal field theories (CFTs)! We are used to physical systems that are invariant under translations and rotations. Imagine a system which is also invariant under scale transformations. Such a system is described by a conformal field theory. Remarkably, many physical systems admit such a description and conformal field theory is ubiquitous in our current theoretical understanding of nature.
Oxford Mathematician Dan Ciubotaru talks about his recent research in Representation Theory.
Oxford Mathematicians Dominic Vella and Finn Box together with colleague Alfonso Castrejón-Pita from Engineering Science in Oxford and Maxime Inizan from MIT have won the annual video competition run by the UK Fluids Network. Here they describe their work and the film.
Oxford Mathematician Ali El Kaafarani explains how mathematics is tackling the issue of post-quantum digital security.
"Quantum computers are on their way to us, not from a galaxy far far away; they are literally right across the road from us in the Physics Department of Oxford University.
Oxford Mathematician Yuuji Tanaka describes his part in the advances in our understanding of gauge theory.
As recent breaches have demonstrated, security will be one of the major concerns of our digital futures. The collective intelligence of the mathematical community is critical to finding these flaws. A group of Oxford Mathematicians, both researchers and undergraduates, have done just that.
Oxford Mathematician Tom Oliver talks about his research in to the rich mine of mathematical information that are L-functions.