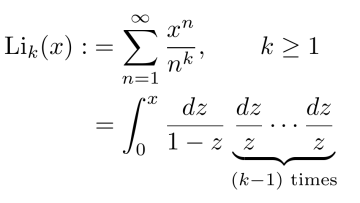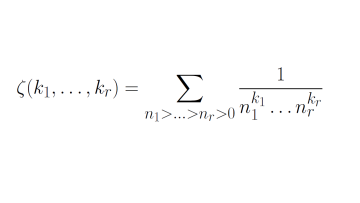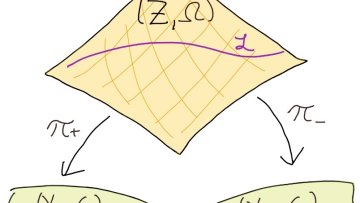
Oxford Mathematician Weijun Xu talks about his exploration of the universal behaviour of large random systems:
"Nature comes with a separation of scales. Systems that have apparently different individual interactions often behave very similarly when looked at from far away. This phenomenon is particularly attractive when randomness is involved.
Mathematics has long played a crucial role in understanding ecological dynamics in a range of ecosystems, from classical models of predators and prey to more recent models of aquatic organisms' interaction with global climate. When we think of ecosystems we usually imagine coral reefs or tropical forests; however, over the past decade a substantial effort has emerged in studying a tiny, yet deadly ecosystem: human tumours. Rather than being a single malignant mass, tumours are living and evolving ecosystems.
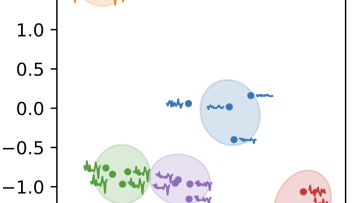
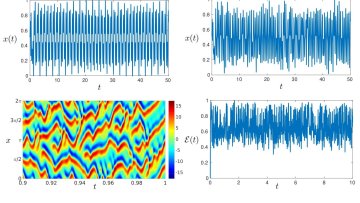
Networks provide powerful tools and methods to understand inter-connected systems. What is the most central element in a system? How does its structure affect a linear or non-linear dynamical system, for instance synchronisation? Is it possible to find groups of elements that play the same role or are densely connected with each other? These are the types of questions that can be answered within the realm of network science. For standard algorithms to be used, however, it is usually expected that the underlying network structure is known.
In 2018, the World Health Organization added “Disease X” to its list of priority diseases, alongside diseases like Ebola virus disease and SARS. Disease X is representative of infectious agents that are not currently known to cause cases in humans. In other words, it denotes the possibility of an epidemic of a disease that we have never seen before.
Consider a function $f: X \rightarrow X$, an initial value $a_0 \in X$, and an iterative sequence $(a_n)$ given by
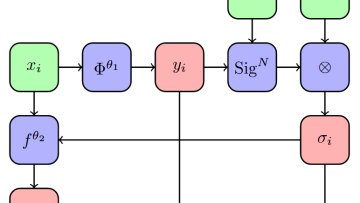
Oxford Mathematician Patrick Kidger talks about his recent work on applying the tools of controlled differential equations to machine learning.
Sequential Data
The changing air pressure at a particular location may be thought of as a sequence in $\mathbb{R}$; the motion of a pen on paper may be thought of as a sequence in $\mathbb{R}^2$; the changes within financial markets may be thought of as a sequence in $\mathbb{R}^d$, with $d$ potentially very large.
Oxford Mathematician Alan Lauder works on elliptic curves and modular forms, and methods for constructing points on the former using the latter. Read more here.
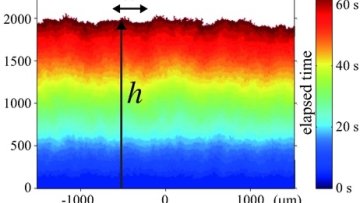
For centuries, engineers have sought to prevent structures from buckling under heavy loads or large impacts, constructing ever larger buildings and safer vehicles. However, recent advances in soft matter are redefining the way we manipulate materials. In particular, an age-old aversion to buckling is being recast in a new light as researchers find that structural instabilities can be harnessed for functionality.
Energy production is arguably one of the most important factors underlying modern civilisation. Energy allows us to inhabit inhospitable parts of the Earth in relative comfort (using heating and air conditioning), create large cities (by efficiently transporting food and pumping water), or maintain our health (providing the energy for water purification). It also connects people by allowing long-distance travel and facilitating digital communication.
Applied mathematics provides a collection of methods to allow scientists and engineers to make the most of experimental data, in order to answer their scientific questions and make predictions. The key link between experiments, understanding, and predictions is a mathematical model: you can find many examples in our case-studies. Experimental data can be used to calibrate a model by inferring the parameters of a real-world system from its observed behaviour.
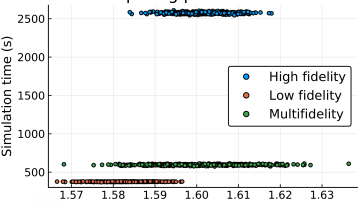
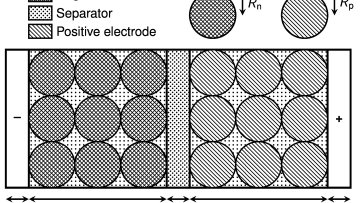
Have you ever wished that the battery on your phone would last longer? That you could charge it up more rapidly? Maybe you have thought about buying an electric vehicle, but were filled with range anxiety – the overwhelming fear that the battery will run out before you reach your destination, leaving you stranded? Oxford Mathematicians are hard at work demonstrating that mathematics may provide the key to help tackle problems faced by the battery industry.
Oxford Mathematician Ma Luo talks about his work on constructing iterated integrals, which generalizes usual integrals, to study elliptic and modular curves.
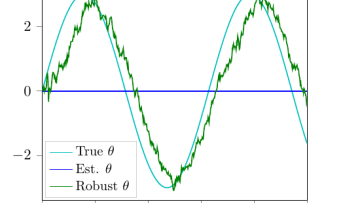
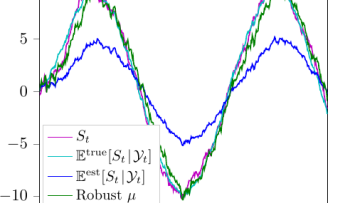
Oxford Mathematicians Andy Allan and Sam Cohen talk about their recent work on estimating with uncertainty.
Oxford Mathematician Nils Matthes talks about trying to understand old numbers using new techniques.
"The Riemann zeta function is arguably one of the most important objects in arithmetic. It encodes deep information about the whole numbers; for example the celebrated Riemann hypothesis, which gives a precise location of its zeros, predicts deep information about the prime numbers. In my research, I am mostly interested in the special values of the Riemann zeta function at integers $k\geq 2$,
Elementary particles in two dimensional systems are not constrained by the fermion-boson alternative. They are so-called "anyons''. Anyon systems are modelled by modular tensor categories, and form an active area of research. Oxford Mathematician André Henriques explains his interest in the question.
Minimal Lagrangians are key objects in geometry, with many connections ranging from classical problems through to modern theoretical physics, but where and how do we find them? Oxford Mathematician Jason Lotay describes some of his research on these questions.
"A classical problem in geometry going back at least to Ancient Greece is the so-called isoperimetric problem: what is the shortest curve in the plane enclosing a given area A? The answer is a circle:
The 1918 Spanish influenza pandemic claimed around fifty million lives worldwide. Interventions were introduced to reduce the spread of the virus, but these were not based on quantitative assessments of the likely effects of different control strategies. One hundred years later, mathematical modelling is routinely used for forecasting and to help plan interventions during outbreaks in populations of humans, animals and plants.
Have you ever picked up a glass to find that the coaster it was resting on remains stuck to the bottom? If so, then you have experienced the ability of fluid to stick two surfaces together. When the bottom of the glass is wetted, for example by accidentally spilling a drink, then this fluid can fill the gap between the glass and coaster. The surface tension of the liquid then provides a pulling force on the coaster that keeps it attached to the glass.
