Systems of differential equations have a key role in biological and chemical models. These models come with parameters that show the model’s dependency on the environmental effects and often have unknown values. Model simulations from observation are desired not to be affected by the values of the parameters. In other words, we would like the parameters to be identifiable from the input-output behaviour of the system.
In mathematics, as in footwear, order matters. Putting on your socks before your shoes produces a different result to putting on your shoes before your socks. This means that the two operations 'putting on socks' and 'putting on shoes' are noncommutative. Noncommutative structures are widespread in mathematics, appearing in subjects ranging from group theory to analysis and differential equations.
We all know that mathematical activity goes on nowadays in a great variety of settings – not just in academia, but across the whole range of industry, education, and beyond. This diversity in mathematics is by no means new, and yet the study of the history of mathematics has often failed to capture it.
Holography is one of a set of powerful tools which theoretical physicists use to understand the fundamental aspects of nature. The holographic principle states that the entire information content of a theory of quantum gravity in some volume is equivalent (or dual) to a theory living at the boundary of the volume without gravity. The boundary degrees of freedom encode all the bulk degrees of freedom and their dynamics and vice versa.
Systems of chemical reactions figure prominently in models throughout the natural sciences. Biology in particular gives rise to hugely complicated reaction networks which, somehow, perform intricate and delicate functions. How do we make sense of these complex networks, and try to understand the relationships between their structure and function?
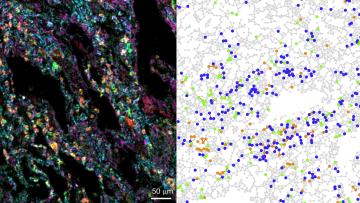
Mathematical modelling played a key role in describing the spread of the COVID-19 pandemic; now a different kind of maths is helping us understand how immune cells interact in the lungs of patients with severe COVID-19.
In a damaged lung with a massive immune cell infiltrate, as seen with severe COVID-19 infection, it can be difficult to figure out which cells are involved in causing lung injury.
For the first several centuries of its existence, an education at the University of Oxford entailed a basic grounding in a range of different subjects, rather than the specialised study of a single discipline. The goal was to turn out well-rounded individuals rather than narrow experts. Nevertheless, the university often tried, wherever possible, to provide advanced instruction in specific areas for those students who were interested.
In this case study we'll highlight new world records, going 23% beyond the Riemann Hypothesis. To explain, we start with the (last digit of) prime numbers: \[ {\color{blue}{\bf 2}}, {\color{green}{\bf3}}, {\color{orange}{\bf5}}, {\color{red}{\bf7}}, 1{\bf1}, 1{\color{green}{\bf3}}, 1{\color{red}{\bf7}}, 1{\color{purple}{\bf9}}, 2{\color{green}{\bf3}},\ldots \] After some thought, we may realize that no such last digit may be even (after ${\color{blue}{\bf 2}}$ itself), else the whole number is even; nor may ${\color{orange}{\bf5}}$ appear again.
During the COVID-19 pandemic, mathematical modelling played a major role in informing public health policy responses. A key question for public health policy makers is whether the introduction of a virus into a population is likely to lead to sustained transmission. This is critical for understanding the epidemic and/or pandemic potential of a novel virus – notably, for example, following the first detected COVID-19 cases in Wuhan, China.
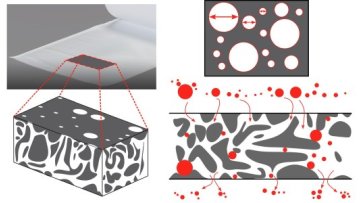
Many modern challenges facing industry and government relate to cleaning: removing pollution from water or industrial waste gases, or decontaminating the environment after chemical spills. Mathematicians in Oxford are collaborating with various industrial and government partners to model specific cleaning challenges, deepening understanding of these processes and working towards optimal cleaning solutions.
Researchers at the University of Oxford’s Botanic Garden and in Oxford Mathematics have shown that the shape, size and geometry of carnivorous pitcher plants determines the type of prey they trap. The results have been published today in the Proceedings of the National Academy of Sciences (PNAS).
In many modern applications, a key bottleneck is the solution of a matrix problem of the form Ax=b where A is a large matrix. In numerical weather prediction, such systems arise as a sub-problem within data assimilation algorithms. In this setting, finding the most likely initial condition with which to initialise a forecast is equivalent to finding the (approximate) solution x.
Symmetry underpins all physics research. We look for fundamental and beautiful patterns to describe and explain the laws of nature. One way of explaining symmetry is to ask: "what is the full set of operations I can do to my real-world experiment or abstract theory written on paper that doesn't change any physical measurements or predictions?'' There are simple symmetries we are perhaps already familiar with. For example, lab-based physics experiments usually don't care if you wait an hour to do the experiment or if you rotate your apparatus by 90 degrees.
Inspired by jumping insects, Oxford Mathematicians have helped develop a miniature robot capable of leaping more than 40 times its body length - equivalent to a human jumping up to the 20th floor of a building. The innovation could be a major step forward in developing miniature robots for a wide range of applications.
---
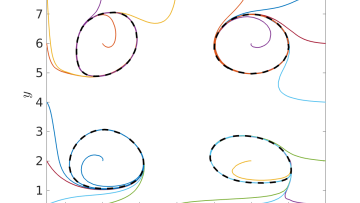
In 1900, David Hilbert posed his list of 23 mathematical problems. While some of them have been resolved in subsequent years, a few of his challenging questions remain unanswered to this day. One of them is Hilbert's 16th problem, which asks questions about the number of limit cycles that a system of ordinary differential equations can have. Such equations appear in modelling real-world systems in biological, chemical or physical applications, where their solutions are functions of time.
What do quantum mechanics, genetics, economic choice theory and ant behaviour have in common? Naturally, the first answer is that they have nothing at all to do with each other! Nonetheless, finding bridges between different fields is the bread and butter of the field of complex systems, an offshoot of statistical physics and other fields.
Algebraic topology is the study of the continuous shape of spaces by the discrete means of algebra. The beginning of modern algebraic topology can be traced back to an insight of Pontryagin in the 1930s which relates the global smooth geometry of manifolds to algebraic invariants associated to the local symmetries of those manifolds - this relation converts something smooth and geometric (called a manifold, potentially endowed with further structure) to something algebraic that can be written down with symbols and formulas, i.e.